Homotopy extension property
In mathematics, in the area of algebraic topology, the homotopy extension property indicates which homotopies defined on a subspace can be extended to a homotopy defined on a larger space.
Definition
Let 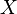 be a topological space, and let
be a topological space, and let  .
We say that the pair
.
We say that the pair  has the homotopy extension property if, given a homotopy
has the homotopy extension property if, given a homotopy 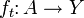 and a map
and a map 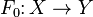 such that
such that  , there exists an extension of
, there exists an extension of  to a homotopy
to a homotopy 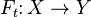 such that
such that
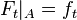 . [1]
. [1]
That is, the pair  has the homotopy extension property if any map
has the homotopy extension property if any map
 can be extended to a map
can be extended to a map 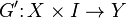 (i.e.
(i.e. 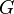 and
and  agree on their common domain).
agree on their common domain).
If the pair has this property only for a certain codomain 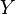 , we say that
, we say that  has the homotopy extension property with respect to
has the homotopy extension property with respect to 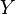 .
.
Visualisation
The homotopy extension property is depicted in the following diagram

If the above diagram (without the dashed map) commutes, which is equivalent to the conditions above, then there exists a map 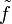 which makes the diagram commute. By currying, note that a map
which makes the diagram commute. By currying, note that a map 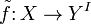 is the same as a map
is the same as a map 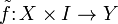 .
.
Also compare this to the visualization of the homotopy lifting property.
Properties
- If
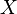 is a cell complex and
is a cell complex and  is a subcomplex of
is a subcomplex of 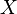 , then the pair
, then the pair  has the homotopy extension property.
has the homotopy extension property.
- A pair
 has the homotopy extension property if and only if
has the homotopy extension property if and only if 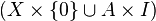 is a retract of
is a retract of 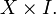
Other
If 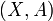 has the homotopy extension property, then the simple inclusion map
has the homotopy extension property, then the simple inclusion map  is a cofibration.
is a cofibration.
In fact, if you consider any cofibration 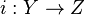 , then we have that
, then we have that  is homeomorphic to its image under
is homeomorphic to its image under 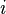 . This implies that any cofibration can be treated as an inclusion map, and therefore it can be treated as having the homotopy extension property.
. This implies that any cofibration can be treated as an inclusion map, and therefore it can be treated as having the homotopy extension property.
See also
References
- ↑ A. Dold, Lectures on Algebraic Topology, pp. 84, Springer ISBN 3-540-58660-1
- Hatcher, Allen (2002). Algebraic Topology. Cambridge University Press. ISBN 0-521-79540-0.