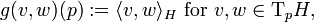Hilbert manifold
In mathematics, a Hilbert manifold is a manifold modeled on Hilbert spaces. Thus it is a separable Hausdorff space in which each point has a neighbourhood homeomorphic to an infinite dimensional Hilbert space. The concept of a Hilbert manifold provides a possibility of extending the theory of manifolds to infinite-dimensional setting. Analogously to the finite-dimensional situation, one can define a differentiable Hilbert manifold by considering a maximal atlas in which the transition maps are differentiable.
Properties
Many basic constructions of the manifold theory, such as the tangent space of a manifold and a tubular neighbourhood of a submanifold (of finite codimension) carry over from the finite dimensional situation to the Hilbert setting with little change. However, in statements involving maps between manifolds, one often has to restrict consideration to Fredholm maps, i.e. maps whose differential at every point is Fredholm. The reason for this is that Sard's lemma holds for Fredholm maps, but not in general. Notwithstanding this difference, Hilbert manifolds have several very nice properties.
- Kuiper's theorem: If X is a compact topological space or has the homotopy type of a CW-Complex then every (real or complex) Hilbert space bundle over X is trivial. In particular, every Hilbert manifold is parallelizable.
- Every smooth Hilbert manifold can be smoothly embedded onto an open subset of the model Hilbert space.
- Every homotopy equivalence between two Hilbert manifolds is homotopic to a diffeomorphism. In particular every two homotopy equivalent Hilbert manifolds are already diffeomorphic. This stands in contrast to lens spaces and exotic spheres, which demonstrate that in the finite-dimensional situation, homotopy equivalence, homeomorphism, and diffeomorphism of manifolds are distinct properties.
- Although Sard's Theorem does not hold in general, every continuous map f : X → Rn from a Hilbert manifold can be arbitrary closely approximated by a smooth map g : X → Rn which has no critical points
Examples
- Any Hilbert space H is a Hilbert manifold with a single global chart given by the identity function on H. Moreover, since H is a vector space, the tangent space TpH to H at any point p ∈ H is canonically isomorphic to H itself, and so has a natural inner product, the "same" as the one on H. Thus, H can be given the structure of a Riemannian manifold with metric
- where ⟨·, ·⟩H denotes the inner product in H.
- Similarly, any open subset of a Hilbert space is a Hilbert manifold and a Riemannian manifold under the same construction as for the whole space.
- There are several mapping spaces between manifolds which can be viewed as Hilbert spaces by only considering maps of suitable Sobolev class. For example we can consider the space LM of all H1 maps from the unit circle S1 into a manifold M. This can be topologized via the compact open topology as a subspace of the space of all continuous mappings from the circle to M, i.e. the free loop space of M. The Sobolev kind mapping space LM described above is homotopy equivalent to the free loop space. This makes it suited to the study of algebraic topology of the free loop space, especially in the field of string topology. We can do an analogous Sobolev construction for the loop space, making it a codimension d Hilbert submanifold of LM, where d is the dimension of M.
See also
References
- Klingenberg, Wilhelm (1982), Riemannian Geometry, Berlin: W. de Gruyter, ISBN 978-3-11-008673-7. Contains a general introduction to Hilbert manifolds and many details about the free loop space.
- Lang, Serge (1995), Differential and Riemannian Manifolds, New York: Springer, ISBN 978-0387943381. Another introduction with more differential topology.
- N. Kuiper, The homotopy type of the unitary group of Hilbert spaces", Topology 3, 19-30
- J. Eells, K. D. Elworthy, "On the differential topology of Hilbert manifolds", Global analysis. Proceedings of Symposia in Pure Mathematics, Volume XV 1970, 41-44.
- J. Eells, K. D. Elworthy, "Open embeddings of certain Banach manifolds", Annals of Mathematics 91 (1970), 465-485
- D. Chataur, "A Bordism Approach to String Topology", preprint http://arxiv.org/abs/math.at/0306080
External links
- Hilbert manifold at the Manifold Atlas