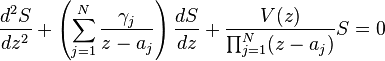Heine–Stieltjes polynomials

For the orthogonal polynomials, see Stieltjes-Wigert polynomial. For the polynomials associated to a family of orthogonal polynomials, see Stieltjes polynomials.

In mathematics, the Heine–Stieltjes polynomials or Stieltjes polynomials, introduced by T. J. Stieltjes (1885), are polynomial solutions of a second-order Fuchsian equation, a differential equation all of whose singularities are regular. The Fuchsian equation has the form

\frac{d^2 S}{dz^2}+\left(\sum _{j=1}^N \frac{\gamma _j}{z - a_j} \right) \frac{dS}{dz} + \frac{V(z)}{\prod _{j=1}^N (z - a_j)}S = 0

for some polynomial V(z) of degree at most N − 2, and if this has a polynomial solution S then V is called a Van Vleck polynomial (after Edward Burr Van Vleck) and S is called a Heine–Stieltjes polynomial.

Heun polynomials are the special cases of Stieltjes polynomials when the differential equation has four singular points.

References

Marden, Morris (1931), "On Stieltjes Polynomials", Transactions of the American Mathematical Society (Providence, R.I.: American Mathematical Society) 33 (4): 934–944, doi:10.2307/1989516, ISSN 0002-9947, JSTOR 1989516
Sleeman, B. D.; Kuznetzov, V. B. (2010), "Stieltjes Polynomials", in Olver, Frank W. J.; Lozier, Daniel M.; Boisvert, Ronald F.; Clark, Charles W., NIST Handbook of Mathematical Functions, Cambridge University Press, ISBN 978-0521192255, MR 2723248
Stieltjes, T. J. (1885), "Sur certains polynômes qui vérifient une équation différentielle linéaire du second ordre et sur la theorie des fonctions de Lamé", Acta Mathematica 6 (1): 321–326, doi:10.1007/BF02400421

This article is issued from Wikipedia - version of the Wednesday, January 27, 2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.