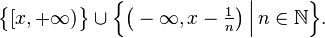Lower limit topology
In mathematics, the lower limit topology or right half-open interval topology is a topology defined on the set R of real numbers; it is different from the standard topology on R (generated by the open intervals) and has a number of interesting properties. It is the topology generated by the basis of all half-open intervals [a,b), where a and b are real numbers.
The resulting topological space, sometimes written Rl and called the Sorgenfrey line after Robert Sorgenfrey, often serves as a useful counterexample in general topology, like the Cantor set and the long line. The product of Rl with itself is also a useful counterexample, known as the Sorgenfrey plane.
In complete analogy, one can also define the upper limit topology, or left half-open interval topology.
Properties
- The lower limit topology is finer (has more open sets) than the standard topology on the real numbers (which is generated by the open intervals). The reason is that every open interval can be written as a countably infinite union of half-open intervals.
- For any real
 and
and 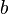 , the interval
, the interval  is clopen in
is clopen in 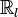 (i.e., both open and closed). Furthermore, for all real
(i.e., both open and closed). Furthermore, for all real  , the sets
, the sets 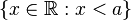 and
and 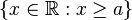 are also clopen. This shows that the Sorgenfrey line is totally disconnected.
are also clopen. This shows that the Sorgenfrey line is totally disconnected.
- Any compact subset of
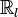 must be an at most countable set. To see this, consider a non-empty compact subset
must be an at most countable set. To see this, consider a non-empty compact subset 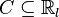 . Fix an
. Fix an 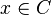 , consider the following open cover of
, consider the following open cover of  :
:
- Since
 is compact, this cover has a finite subcover, and hence there exists a real number
is compact, this cover has a finite subcover, and hence there exists a real number  such that the interval
such that the interval ![(a(x), x]](../I/m/689da822e7cd1324db5892e7ffb7e113.png) contains no point of
contains no point of  apart from
apart from  . This is true for all
. This is true for all 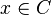 . Now choose a rational number
. Now choose a rational number ![q(x) \in (a(x), x]\cap\mathbb{Q}](../I/m/47a623c7b59e2385c928373e137bacdb.png) . Since the intervals
. Since the intervals ![(a(x), x]](../I/m/689da822e7cd1324db5892e7ffb7e113.png) , parametrized by
, parametrized by 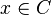 , are pairwise disjoint, the function
, are pairwise disjoint, the function 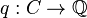 is injective, and so
is injective, and so  is at most countable.
is at most countable.
- The name "lower limit topology" comes from the following fact: a sequence (or net)
 in
in 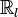 converges to the limit
converges to the limit  iff it "approaches
iff it "approaches  from the right", meaning for every
from the right", meaning for every  there exists an index
there exists an index  such that
such that 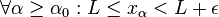 . The Sorgenfrey line can thus be used to study right-sided limits: if
. The Sorgenfrey line can thus be used to study right-sided limits: if 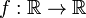 is a function, then the ordinary right-sided limit of
is a function, then the ordinary right-sided limit of  at
at  (when the codomain carry the standard topology) is the same as the usual limit of
(when the codomain carry the standard topology) is the same as the usual limit of  at
at  when the domain is equipped with the lower limit topology and the codomain carries the standard topology.
when the domain is equipped with the lower limit topology and the codomain carries the standard topology.
- In terms of separation axioms,
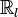 is a perfectly normal Hausdorff space.
is a perfectly normal Hausdorff space.
- In terms of countability axioms,
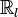 is first-countable and separable, but not second-countable.
is first-countable and separable, but not second-countable.
- In terms of compactness properties,
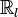 is Lindelöf and paracompact, but not σ-compact nor locally compact.
is Lindelöf and paracompact, but not σ-compact nor locally compact.
-
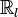 is not metrizable, since separable metric spaces are second-countable. However, the topology of a Sorgenfrey line is generated by a premetric.
is not metrizable, since separable metric spaces are second-countable. However, the topology of a Sorgenfrey line is generated by a premetric.
-
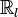 is a Baire space .
is a Baire space .
References
- Steen, Lynn Arthur; Seebach, J. Arthur Jr. (1995) [1978], Counterexamples in Topology (Dover reprint of 1978 ed.), Berlin, New York: Springer-Verlag, ISBN 978-0-486-68735-3, MR 507446