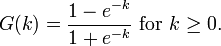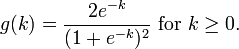Half-logistic distribution

Half-logistic distribution
Probability density function
Cumulative distribution function
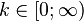Support	$k \in [0;\infty)\!$
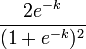PDF	$\frac{2 e^{-k}}{(1+e^{-k})^2}\!$
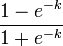CDF	$\frac{1-e^{-k}}{1+e^{-k}}\!$
Mean	$\log_e(4)=1.386\ldots$
Median	$\log_e(3)=1.0986\ldots$
Mode	0
Variance	$\pi^2/3-(\log_e(4))^2=1.368\ldots$

In probability theory and statistics, the half-logistic distribution is a continuous probability distribution—the distribution of the absolute value of a random variable following the logistic distribution. That is, for

X = |Y| \!

where Y is a logistic random variable, X is a half-logistic random variable.

Specification

Cumulative distribution function

The cumulative distribution function (cdf) of the half-logistic distribution is intimately related to the cdf of the logistic distribution. Formally, if F(k) is the cdf for the logistic distribution, then G(k) = 2F(k) − 1 is the cdf of a half-logistic distribution. Specifically,

G(k) = \frac{1-e^{-k}}{1+e^{-k}} \mbox{ for } k\geq 0. \!

Probability density function

Similarly, the probability density function (pdf) of the half-logistic distribution is g(k) = 2f(k) if f(k) is the pdf of the logistic distribution. Explicitly,

g(k) = \frac{2 e^{-k}}{(1+e^{-k})^2} \mbox{ for } k\geq 0. \!

References

George, Olusengun; Meenakshi Devidas (1992). "Some Related Distributions". In N. Balakrishnan. Handbook of the Logistic Distribution. New York: Marcel Dekker, Inc. pp. 232–234. ISBN 0-8247-8587-8.
Olapade, A.K. (February 2003). "On Characterizations of the Half-Logistic Distribution" (PDF). InterStat (2).

Probability distributions

Discrete univariate with finite support

Benford Bernoulli Beta-binomial binomial categorical hypergeometric Poisson binomial Rademacher discrete uniform Zipf Zipf–Mandelbrot

Discrete univariate with infinite support

beta negative binomial Borel Conway–Maxwell–Poisson discrete phase-type Delaporte extended negative binomial Gauss–Kuzmin geometric logarithmic negative binomial parabolic fractal Poisson Skellam Yule–Simon zeta

Continuous univariate supported on a bounded interval, e.g. [0,1]

Arcsine ARGUS Balding–Nichols Bates Beta Beta rectangular Irwin–Hall Kumaraswamy logit-normal Noncentral beta raised cosine Reciprocal Triangular U-quadratic uniform Wigner semicircle

[[List of probability distributions#Supported_on_semi-infinite_intervals.2C_usually_.5B0.2C.E2.88.9E.29|Continuous univariate supported on a semi-infinite interval, usually [0,∞)]]

Continuous univariate supported on the whole real line (−∞, ∞)

Cauchy exponential power Fisher's z generalized normal generalized hyperbolic geometric stable Gumbel Holtsmark hyperbolic secant Johnson S_U Landau Laplace Asymmetric Laplace Linnik logistic noncentral t normal (Gaussian) normal-inverse Gaussian skew normal slash stable Student's t type-1 Gumbel Tracy–Widom variance-gamma Voigt

Continuous univariate with support whose type varies

generalized extreme value generalized Pareto Tukey lambda q-Gaussian q-exponential q-Weibull shifted log-logistic

Mixed continuous-discrete univariate distributions

rectified Gaussian

Multivariate (joint)

Discrete Ewens multinomial Dirichlet-multinomial negative multinomial Continuous Dirichlet Generalized Dirichlet multivariate normal Multivariate stable multivariate Student normal-scaled inverse gamma normal-gamma Matrix-valued inverse matrix gamma inverse-Wishart matrix normal matrix t matrix gamma normal-inverse-Wishart normal-Wishart Wishart

Directional

Univariate (circular) directional Circular uniform univariate von Mises wrapped normal wrapped Cauchy wrapped exponential wrapped asymmetric Laplace wrapped Lévy Bivariate (spherical) Kent Bivariate (toroidal) bivariate von Mises Multivariate von Mises–Fisher Bingham

Degenerate and singular

Degenerate discrete degenerate Dirac delta function Singular Cantor

Families

Circular compound Poisson elliptical exponential natural exponential location-scale maximum entropy mixture Pearson Tweedie wrapped

This article is issued from Wikipedia - version of the Thursday, February 04, 2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.