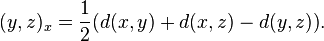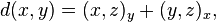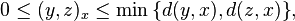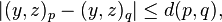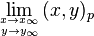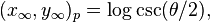Gromov product
In mathematics, the Gromov product is a concept in the theory of metric spaces named after the mathematician Mikhail Gromov. The Gromov product can also be used to define δ-hyperbolic metric spaces in the sense of Gromov.
Definition
Let (X, d) be a metric space and let x, y, z ∈ X. Then the Gromov product of y and z at x, denoted (y, z)x, is defined by
Motivation
Given three points x, y, z in the metric space X, by the triangle inequality there exist non negative numbers a, b, c such that 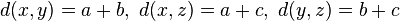 . Then the Gromov products are
. Then the Gromov products are 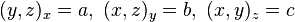 . In the case that the points x, y, z are the outer nodes of a tripod then these Gromov products are the lengths of the edges.
. In the case that the points x, y, z are the outer nodes of a tripod then these Gromov products are the lengths of the edges.
.svg.png)
In Euclidean space, the Gromov product (y, z)x equals the distance between x and the point where the inscribed circle of the triangle xyz touches the edge xy.
Properties
- The Gromov product is symmetric: (y, z)x = (z, y)x.
- The Gromov product degenerates at the endpoints: (y, z)y = (y, z)z = 0.
- For any points p, q, x, y and z,
Points at infinity
Consider hyperbolic space Hn. Fix a base point p and let 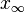 and
and 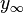 be two distinct points at infinity. Then the limit
be two distinct points at infinity. Then the limit
exists and is finite, and therefore can be considered as a generalized Gromov product. It is actually given by the formula
where  is the angle between the geodesic rays
is the angle between the geodesic rays 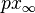 and
and 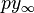 .[1]
.[1]
δ-hyperbolic spaces and divergence of geodesics
The Gromov product can be used to define δ-hyperbolic spaces in the sense of Gromov.: (X, d) is said to be δ-hyperbolic if, for all p, x, y and z in X,
In this case. Gromov product measures how long geodesics remain close together. Namely, if x, y and z are three points of a δ-hyperbolic metric space then the initial segments of length (y, z)x of geodesics from x to y and x to z are no further than 2δ apart (in the sense of the Hausdorff distance between closed sets).
References
- Kapovich, Ilya; Benakli, Nadia (2002). "Boundaries of hyperbolic groups". Combinatorial and geometric group theory (New York, 2000/Hoboken, NJ, 2001). Contemp. Math. 296. Providence, RI: Amer. Math. Soc. pp. 39–93. MR 1921706.
- Väisälä, Jussi (2004). "Gromov hyperbolic spaces" (PDF). Retrieved 2007-08-28.