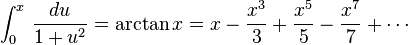Gregory's series

Gregory's series, also known as the Madhava–Gregory series or Leibniz's series, is an infinite Taylor series expansion of the inverse tangent function. It was discovered by the Indian mathematician Madhava of Sangamagrama (1350- 1410) (Gupta 1973) and independently rediscovered and published in 1668 by James Gregory. It was rediscovered a few years later by Gottfried Leibniz, who obtained the Leibniz formula for π as the special case x = 1 of the Gregory series.[1]

Definition

Gregory's series is defined as below:

\int_0^x \, \frac{du}{1+u^2} = \arctan x = x - \frac{x^3}{3} + \frac{x^5}{5} - \frac{x^7}{7} + \cdots

Compare with the series for sine, which is similar but has factorials in the denominator.

History

In 1668 Gregory published two works. One of them, Geometriae pars universalis (The Universal Part of Geometry), was published in Padua; the other, Exercitationes geometrica (Geometrical Exercises), at London. He divided mathematics into "general" and "special" groups of theorems. He discovered the Taylor series more than forty years before Brook Taylor published it. The series for arctan x bears his name.

See also

References

  1. ↑ "Gregory Series". Wolfram Math World. Retrieved 26 July 2012.
This article is issued from Wikipedia - version of the Wednesday, May 13, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.