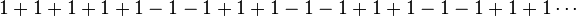Grandi's series
In mathematics, the infinite series 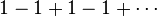 , also written
, also written
is sometimes called Grandi's series, after Italian mathematician, philosopher, and priest Guido Grandi, who gave a memorable treatment of the series in 1703. It is a divergent series, meaning that it lacks a sum in the usual sense. On the other hand, its Cesàro sum is 1/2.
Proof through integration
To prove using integration, first we consider the indefinite integral of 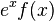 with respect to
with respect to  for any function
for any function  by repeating integration by parts, with
by repeating integration by parts, with  and
and 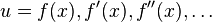 :
:
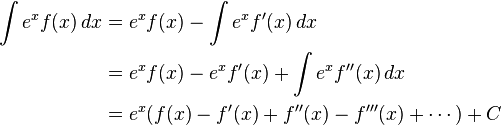
Here,  is the constant of integration.
is the constant of integration.
By letting 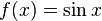 or
or  , we will get a result relevant to solving the Grandi series
, we will get a result relevant to solving the Grandi series  :
:
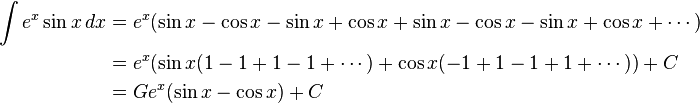
We can also evaluate the integral using integration by parts with  and
and  . We treat the integral as a function
. We treat the integral as a function  and realize that we have the same integral on both sides of the equation and add
and realize that we have the same integral on both sides of the equation and add  to both sides and divide by two. Symbolically:
to both sides and divide by two. Symbolically:
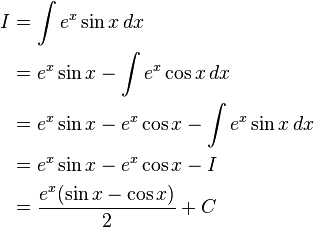
Comparing the results of evaluating the integral using both methods 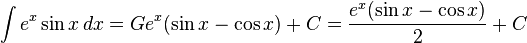 and thus
and thus  .
.
Proof through 1 / x series
The series:

Is fairly easy to prove. First, multiply everything by x. On the left side, this makes 1, and on the right side we'll represent x as (x - 1) + 1. Multiply the series by (x - 1) and 1 separately and add the two together.

All terms except 1 cancel out, leaving:

Applying this series to 2 gives:
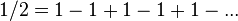
Unrigorous Methods
One obvious method to attack the series
- 1 − 1 + 1 − 1 + 1 − 1 + 1 − 1 + ...
is to treat it like a telescoping series and perform the subtractions in place:
- (1 − 1) + (1 − 1) + (1 − 1) + ... = 0 + 0 + 0 + ... = 0.
On the other hand, a similar bracketing procedure leads to the apparently contradictory result
- 1 + (−1 + 1) + (−1 + 1) + (−1 + 1) + ... = 1 + 0 + 0 + 0 + ... = 1.
Thus, by applying parentheses to Grandi's series in different ways, one can obtain either 0 or 1 as a "value". (Variations of this idea, called the Eilenberg–Mazur swindle, are sometimes used in knot theory and algebra.)
Treating Grandi's series as a divergent geometric series we may use the same algebraic methods that evaluate convergent geometric series to obtain a third value:
- S = 1 − 1 + 1 − 1 + ..., so
- 1 − S = 1 − (1 − 1 + 1 − 1 + ...) = 1 − 1 + 1 − 1 + ... = S,
resulting in S = 1/2. The same conclusion results from calculating −S, subtracting the result from S, and solving 2S = 1.[1]
The above manipulations do not consider what the sum of a series actually means. Still, to the extent that it is important to be able to bracket series at will, and that it is more important to be able to perform arithmetic with them, one can arrive at two conclusions:
In fact, both of these statements can be made precise and formally proven, but only using well-defined mathematical concepts that arose in the 19th century. After the late 17th-century introduction of calculus in Europe, but before the advent of modern rigor, the tension between these answers fueled what has been characterized as an "endless" and "violent" dispute between mathematicians.[3][4]
The formula for the sum to infinity of a Geometric series is  , although the derivation for the sum is only valid when
, although the derivation for the sum is only valid when 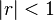 (as with the modulus of r less than one then the terms of the series will decay to zero as n increases and the series will converge) the formula still works for r= -1.
In the case of
(as with the modulus of r less than one then the terms of the series will decay to zero as n increases and the series will converge) the formula still works for r= -1.
In the case of 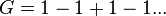 with
with  and
and  , the sum to infinity of G is
, the sum to infinity of G is  and
and 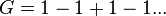 converges to
converges to 
Early ideas
Divergence
In modern mathematics, the sum of an infinite series is defined to be the limit of the sequence of its partial sums, if it exists. The sequence of partial sums of Grandi's series is 1, 0, 1, 0, ..., which clearly does not approach any number (although it does have two accumulation points at 0 and 1). Therefore, Grandi's series is divergent.
It can be shown that it is not valid to perform many seemingly innocuous operations on a series, such as reordering individual terms, unless the series is absolutely convergent. Otherwise these operations can alter the result of summation.[5] Further, the terms of Grandi's series can be rearranged to have its accumulation points at any interval of two or more consecutive integer numbers, not only 0 or 1. For instance, the series
(in which, after five initial +1 terms, the terms alternate in pairs of +1 and −1 terms) is a permutation of Grandi's series in which each value in the rearranged series corresponds to a value that is at most four positions away from it in the original series; its accumulation points are 3, 4, and 5.
Education
Summability
Related problems
See also
- 1 − 1 + 2 − 6 + 24 − 120 + · · ·
- 1 − 2 + 3 − 4 + · · ·
- 1 + 2 + 3 + 4 + · · ·
- Ramanujan summation
- Cesàro summation
- Thomson's lamp
Notes
- 1 2 Devlin p.77
- 1 2 Davis p.152
- ↑ Kline 1983 p.307
- ↑ Knopp p.457
- ↑ Protter, Murray H.; Morrey, Charles B., Jr. (1991), A First Course in Real Analysis, Undergraduate Texts in Mathematics, Springer, p. 249, ISBN 9780387974378.
References
- Davis, Harry F. (May 1989). Fourier Series and Orthogonal Functions. Dover. ISBN 0-486-65973-9.
- Devlin, Keith (1994). Mathematics, the science of patterns: the search for order in life, mind, and the universe. Scientific American Library. ISBN 0-7167-6022-3.
- Kline, Morris (November 1983). "Euler and Infinite Series". Mathematics Magazine 56 (5): 307–314. doi:10.2307/2690371. JSTOR 2690371.
- Knopp, Konrad (1990) [1922]. Theory and Application of Infinite Series. Dover. ISBN 0-486-66165-2.
- Hobson, E. W. (1907). The theory of functions of a real variable and the theory of Fourier's series. The University of Michigan Historical Mathematics Collection: Cambridge University Press. section 331.
- Whittaker, E. T.; Watson, G. N. (1962). A course of modern analysis (4th, reprinted ed.). Cambridge University Press.
 2.1.
2.1.
External links
- One minus one plus one minus one - Numberphile, Grandi's series
| ||||||||||||||||||||||||||||||||||