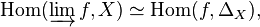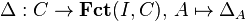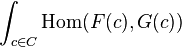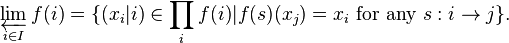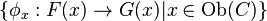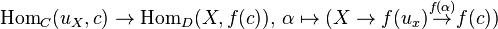Glossary of category theory
This is a glossary of properties and concepts in category theory in mathematics.[1]
Especially for higher categories, the concepts from algebraic topology are also used in the category theory. For that see also glossary of algebraic topology.
The notations used throughout the article are:
- [n] = { 0, 1, 2, …, n }, which is viewed as a category (by writing
 .)
.) - Cat, the category of (small) categories, where the objects are categories (which are small with respect to some universe) and the morphisms natural transformations.
- Fct(C, D), the functor category: the category of functors from a category C to a category D.
- Set, the category of (small) sets.
- sSet, the category of simplicial sets.
A
- abelian
- A category is abelian if it has a zero object, it has all pullbacks and pushouts, and all monomorphisms and epimorphisms are normal.
- additive
- A category is additive if it is preadditive and admits all finitary biproducts.
- adjunction
- An adjunction (also called an adjoint pair) is a pair of functors F: C → D, G: D → C such that there is a "natural" bijection
 ;
;
 of bifunctors (which are contravariant in the first variable.)
of bifunctors (which are contravariant in the first variable.) - amnestic
- A functor is amnestic if it has the property: if k is an isomorphism and F(k) is an identity, then k is an identity.
B
- balanced
- A category is balanced if every bimorphism is an isomorphism.
- bifunctor
- A bifunctor from a pair of categories C and D to a category E is a functor C × D → E. For example, for any category C,
 is a bifunctor from Cop and C to Set.
is a bifunctor from Cop and C to Set. - bimorphism
- A bimorphism is a morphism that is both an epimorphism and a monomorphism.
C
- cartesian closed
- A category is cartesian closed if it has a terminal object and that any two objects have a product and exponential.
- cartesian morphism
- 1. Given a functor π: C → D (e.g., a prestack over schemes), a morphism f: x → y in C is π-cartesian if, for each object z in C, each morphism g: z → y in C and each morphism v: π(z) → π(x) in D such that π(g) = π(f) ∘ v, there exists a unique morphism u: z → x such that π(u) = v and g = f ∘ u.
- 2. Given a functor π: C → D (e.g., a prestack over rings), a morphism f: x → y in C is π-coCartesian if, for each object z in C, each morphism g: x → z in C and each morphism v: π(y) → π(z) in D such that π(g) = v ∘ π(f), there exists a unique morphism u: y → z such that π(u) = v and g = u ∘ f. (In short, f is the dual of a π-cartesian morphism.)
- Cartesian square
- A commutative diagram that is isomorphic to the diagram given as a fiber product.
- category
- A category consists of the following data
- A class of objects,
- For each pair of objects X, Y, a set
 , whose elements are called morphisms from X to Y,
, whose elements are called morphisms from X to Y, - For each triple of objects X, Y, Z, a map (called composition)
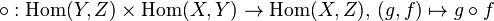 ,
,
- For each object X, an identity morphism
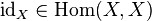
subject to the conditions: for any morphisms
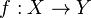 ,
, 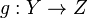 and
and 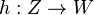 ,
, and
and 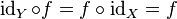 .
.
 if and only if
if and only if  ; the associativity of composition means transitivity.
; the associativity of composition means transitivity. - classifying space
- The classifying space of a category C is the geometric realization of the nerve of C.
- co-
- Often used synonymous with op-; for example, a colimit refers to an op-limit in the sense that it is a limit in the opposite category. But there might be a distinction; for example, an op-fibration is not the same thing as a cofibration.
- coend
- The coend of a functor
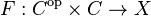 is the dual of the end of F and is denoted by
is the dual of the end of F and is denoted by
 .
.
For example, if R is a ring, M a right R-module and N a left R-module, then the tensor product of M and N is
- coequalizer
- The coequalizer of a pair of morphisms
 is the colimit of the pair. It is the dual of an equalizer.
is the colimit of the pair. It is the dual of an equalizer. - comma
- Given functors
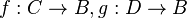 , the comma category
, the comma category  is a category where (1) the objects are morphisms
is a category where (1) the objects are morphisms 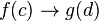 and (2) a morphism from
and (2) a morphism from 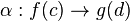 to
to 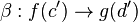 consists of
consists of  and
and 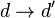 such that
such that 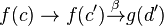 is
is 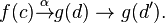 For example, if f is the identity functor and g is the constant functor with a value b, then it is the slice category of B over an object b.
For example, if f is the identity functor and g is the constant functor with a value b, then it is the slice category of B over an object b. - complete
- A category is complete if all small limits exist.
- concrete
- A concrete category C is a category such that there is a faithful functor from C to Set; e.g., Vec, Grp and Top.
- cone
- A cone is a way to express the universal property of a colimit (or dually a limit). One can show[2] that the colimit
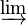 is the left adjoint to the diagonal functor
is the left adjoint to the diagonal functor 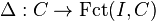 , which sends an object X to the constant functor with value X; that is, for any X and any functor
, which sends an object X to the constant functor with value X; that is, for any X and any functor  ,
,
- connected
- A category is connected if, for each pair of objects x, y, there exists a finite sequence of objects zi such that
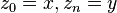 and either
and either 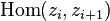 or
or 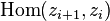 is nonempty for any i.
is nonempty for any i. - constant
- A functor is constant if it maps every object in a category to the same object A and every morphism to the identity on A. Put in another way, a functor
 is constant if it factors as:
is constant if it factors as: 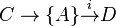 for some object A in D, where i is the inclusion of the discrete category { A }.
for some object A in D, where i is the inclusion of the discrete category { A }. - contravariant functor
- A contravariant functor F from a category C to a category D is a (covariant) functor from Cop to D. It is sometimes also called a presheaf especially when D is Set or the variants. For example, for each function
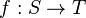 , define
, define
 . With this,
. With this,  is a contravariant functor.
is a contravariant functor. - coproduct
- The coproduct of a family of objects Xi in a category C indexed by a set I is the inductive limit
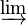 of the functor
of the functor 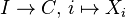 , where I is viewed as a discrete category. It is the dual of the product of the family. For example, a coproduct in Grp is a free product.
, where I is viewed as a discrete category. It is the dual of the product of the family. For example, a coproduct in Grp is a free product.
D
- Day convolution
- Given a group or monoid M, the Day convolution is the tensor product in
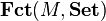 .[4]
.[4] - diagonal functor
- Given categories I, C, the diagonal functor is the functor
 to the natural transformation
to the natural transformation  that is f at each i.
that is f at each i. - discrete
- A category is discrete if each morphism is an identity morphism (of some object). For example, a set can be viewed as a discrete category.
E
- end
- The end of a functor
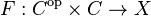 is the limit
is the limit
where
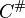 is the category (called the subdivision category of C) whose objects are symbols
is the category (called the subdivision category of C) whose objects are symbols 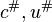 for all objects c and all morphisms u in C and whose morphisms are
for all objects c and all morphisms u in C and whose morphisms are  and
and 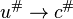 if
if 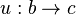 and where
and where  is induced by F so that
is induced by F so that 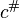 would go to
would go to 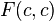 and
and  would go to
would go to  . For example, for functors
. For example, for functors 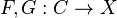 ,
, - empty
- The empty category is a category with no object. It is the same thing as the empty set when the empty set is viewed as a discrete category.
- epimorphism
- A morphism f is an epimorphism if
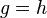 whenever
whenever 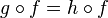 . In other words, f is the dual of a monomorphism.
. In other words, f is the dual of a monomorphism. - equalizer
- The equalizer of a pair of morphisms
 is the limit of the pair. It is the dual of a coequalizer.
is the limit of the pair. It is the dual of a coequalizer. - equivalence
- 1. A functor is an equivalence if it is faithful, full and essentially surjective.
- 2. A morphism in an ∞-category C is an equivalence if it gives an isomorphism in the homotopy category of C.
- equivalent
- A category is equivalent to another category if there is an equivalence between them.
- essentially surjective
- A functor F is called essentially surjective (or isomorphism-dense) if for every object B there exists an object A such that F(A) is isomorphic to B.
F
- faithful
- A functor is faithful if it is injective when restricted to each hom-set.
- fibered category
- A functor π: C → D is said to exhibit C as a category fibered over D if, for each morphism g: x → π(y) in D, there exists a π-cartesian morphism f: x' → y in C such that π(f) = g. If D is the category of affine schemes (say of finite type over some field), then π is more commonly called a prestack. Note: π is often a forgetful functor and in fact the Grothendieck construction implies that every fibered category can be taken to be that form (up to equivalences in a suitable sense).
- fiber product
- Given a category C and a set I, the fiber product over an object S of a family of objects Xi in C indexed by I is the product of the family in the slice category
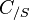 of C over S (provided there are
of C over S (provided there are 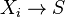 ). The fiber product of two objects X and Y over an object S is denoted by
). The fiber product of two objects X and Y over an object S is denoted by 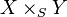 and is also called a Cartesian square.
and is also called a Cartesian square. - filtered
- 1. A filtered category (also called a filtrant category) is a nonempty category with the properties (1) given objects i and j, there are an object k and morphisms i → k and j → k and (2) given morphisms u, v: i → j, there are an object k and a morphism w: j → k such that w ∘ u = w ∘ v. A category I is filtered if and only if, for each finite category J and functor f: J → I, the set
 is nonempty for some object i in I.
is nonempty for some object i in I. - 2. Given a cardinal number π, a category is said to be π-filtrant if, for each category J whose set of morphisms has cardinal number strictly less than π, the set
 is nonempty for some object i in I.
is nonempty for some object i in I. - finite
- A category is finite if it has only finitely many morphisms.
- forgetful functor
- The forgetful functor is, roughly, a functor that loses some of data of the objects; for example, the functor
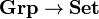 that sends a group to its underlying set and a group homomorphism to itself is a forgetful functor.
that sends a group to its underlying set and a group homomorphism to itself is a forgetful functor. - functor
- Given categories C, D, a functor F from C to D is a structure-preserving map from C to D; i.e., it consists of an object F(x) in D for each object x in C and a morphism F(f) in D for each morphism f in C satisfying the conditions: (1)
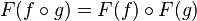 whenever
whenever  is defined and (2)
is defined and (2)  . For example,
. For example,
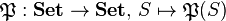 ,
,
 is the power set of S is a functor if we define: for each a function
is the power set of S is a functor if we define: for each a function 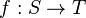 ,
, 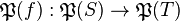 by
by  .
. - full
- 1. A functor is full if it is surjective when restricted to each hom-set.
- 2. A category A is a full subcategory of a category B if the inclusion functor from A to B is full.
G
- generator
- In a category C, a family of objects
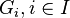 is a system of generators of C if the functor
is a system of generators of C if the functor 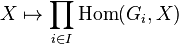 is conservative. Its dual is called a system of cogenerators.
is conservative. Its dual is called a system of cogenerators. - Grothendieck construction
- Given a functor
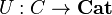 , let DU be the category where the objects are pairs (x, u) consisting of an object x in C and an object u in the category U(x) and a morphism from (x, u) to (y, v) is a pair consisting of a morphism f: x → y in C and a morphism U(f)(u) → v in U(y). The passage from U to DU is then called the Grothendieck construction.
, let DU be the category where the objects are pairs (x, u) consisting of an object x in C and an object u in the category U(x) and a morphism from (x, u) to (y, v) is a pair consisting of a morphism f: x → y in C and a morphism U(f)(u) → v in U(y). The passage from U to DU is then called the Grothendieck construction. - Grothendieck fibration
- A fibered category.
- groupoid
- 1. A category is called a groupoid if every morphism in it is an isomorphism.
- 2. An ∞-category is called an ∞-groupoid if every morphism in it is an equivalence (or equivalently if it is a Kan complex.)
H
- homological dimension
- The homological dimension of an abelian category with enough injectives is the least non-negative intege n such that every object in the category admits an injective resolution of length at most n. The dimension is ∞ if no such integer exists. For example, the homological dimension of ModR with a principal ideal domain R is at most one.
- homotopy category
- See homotopy category. It is closely related to a localization of a category.
I
- identity
- 1. The identity morphism f of an object A is a morphism from A to A such that for any morphisms g with domain A and h with codomain A,
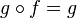 and
and  .
. - 2. The identity functor on a category C is a functor from C to C that sends objects and morphisms to themselves.
- ind-limit
- A colimit (or inductive limit) in
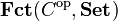 .
. - ∞-category
- An ∞-category C is a simplicial set satisfying the following condition: for each 0 < i < n,
- every map of simplicial sets
 extends to an n-simplex
extends to an n-simplex 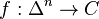
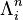 is obtained from Δn by removing the i-th face and the interior (see Kan fibration#Definition). For example, the nerve of a category satisfies the condition and thus can be considered as an ∞-category.
is obtained from Δn by removing the i-th face and the interior (see Kan fibration#Definition). For example, the nerve of a category satisfies the condition and thus can be considered as an ∞-category. - every map of simplicial sets
- initial
- 1. An object A is initial if there is exactly one morphism from A to each object; e.g., empty set in Set.
- 2. An object A in an ∞-category C is initial if
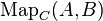 is contractible for each object B in C.
is contractible for each object B in C. - injective
- An object A in an abelian category is injective if the functor
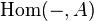 is exact. It is the dual of a projective object.
is exact. It is the dual of a projective object. - internal Hom
- Given a monoidal category (C, ⊗), the internal Hom is a functor
![[-, -]: C^{\text{op}} \times C \to C](../I/m/665216728d9ccd0695f97c3eaa91e7ef.png) such that
such that ![[Y, -]](../I/m/5b3470eefb34241dc0b639892c0786cf.png) is the right adjoint to
is the right adjoint to 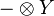 for each object Y in C. For example, the category of modules over a commutative ring R has the internal Hom given as
for each object Y in C. For example, the category of modules over a commutative ring R has the internal Hom given as ![[M, N] = \operatorname{Hom}_R(M, N)](../I/m/96d00d3689593133bb550da1d930d182.png) , the set of R-linear maps.
, the set of R-linear maps. - inverse
- A morphism f is an inverse to a morphism g if
 is defined and is equal to the identity morphism on the codomain of g, and
is defined and is equal to the identity morphism on the codomain of g, and  is defined and equal to the identity morphism on the domain of g. The inverse of g is unique and is denoted by g−1. f is a left inverse to g if
is defined and equal to the identity morphism on the domain of g. The inverse of g is unique and is denoted by g−1. f is a left inverse to g if  is defined and is equal to the identity morphism on the domain of g, and similarly for a right inverse.
is defined and is equal to the identity morphism on the domain of g, and similarly for a right inverse. - isomorphic
- 1. An object is isomorphic to another object if there is an isomorphism between them.
- 2. A category is isomorphic to another category if there is an isomorphism between them.
- isomorphism
- A morphism f is an isomorphism if there exists an inverse of f.
L
- length
- An object in an abelian category is said to have finite length if it has a composition series. The maximum number of proper subobjects in any such composition series is called the length of A.[5]
- limit
- 1. The limit (or projective limit) of a functor
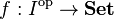 is
is
- 2. The limit
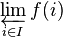 of a functor
of a functor  is an object, if any, in C that satisfies: for any object X in C,
is an object, if any, in C that satisfies: for any object X in C, 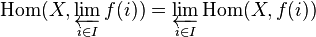 ; i.e., it is an object representing the functor
; i.e., it is an object representing the functor 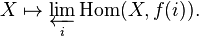
- 3. The colimit (or inductive limit)
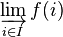 is the dual of a limit; i.e., given a functor
is the dual of a limit; i.e., given a functor  , it satisfies: for any X,
, it satisfies: for any X, 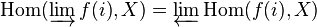 . Explicitly, to give
. Explicitly, to give 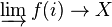 is to give a family of morphisms
is to give a family of morphisms 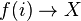 such that for any
such that for any  ,
, 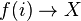 is
is  . Perhaps the simplest example of a colimit is a coequalizer. For another example, take f to be the identity functor on C and suppose
. Perhaps the simplest example of a colimit is a coequalizer. For another example, take f to be the identity functor on C and suppose  exists; then the identity morphism on L corresponds to a compatible family of morphisms
exists; then the identity morphism on L corresponds to a compatible family of morphisms 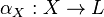 such that
such that 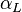 is the identity. If
is the identity. If  is any morphism, then
is any morphism, then  ; i.e., L is a final object of C.
; i.e., L is a final object of C.
- localization of a category
- See localization of a category.
M
- monomorphism
- A morphism f is a monomorphism (also called monic) if
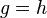 whenever
whenever 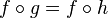 ; e.g., an injection in Set. In other words, f is the dual of an epimorphism.
; e.g., an injection in Set. In other words, f is the dual of an epimorphism.
N
- natural
- 1. A natural transformation is, roughly, a map between functors. Precisely, given a pair of functors F, G from a category C to category D, a natural transformation φ from F to G is a set of morphisms in D
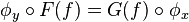 . For example, writing
. For example, writing 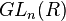 for the group of invertible n-by-n matrices with coefficients in a commutative ring R, we can view
for the group of invertible n-by-n matrices with coefficients in a commutative ring R, we can view 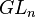 as a functor from the category CRing of commutative rings to the category Grp of groups. Similarly,
as a functor from the category CRing of commutative rings to the category Grp of groups. Similarly,  is a functor from CRing to Grp. Then the determinant det is a natural transformation from
is a functor from CRing to Grp. Then the determinant det is a natural transformation from 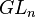 to -*.
to -*. - 2. A natural isomorphism is a natural transformation that is an isomorphism (i.e., admits the inverse).
- nerve
- The nerve functor N is the functor from Cat to sSet given by
![N(C)_n = \operatorname{Hom}_{\mathbf{Cat}}([n], C)](../I/m/4df4fbe7666eb9f444078ed98095d084.png) .
. - normal
- A category is normal if every monic is normal.

The composition is encoded as a 2-simplex.
O
- object
- 1. An object is part of a data defining a category.
- 2. An (adjective) object in a category C is a contravariant functor (or presheaf) from some fixed category corresponding to the "adjective" to C. For example, a simplicial object in C is a contravariant functor from the simplicial category to C and a Γ-object is a pointed contravariant functor from Γ (roughly the pointed category of pointed finite sets) to C provided C is pointed.
- op-fibration
- A functor π:C → D is an op-fibration if, for each object x in C and each morphism g : π(x) → y in D, there is at least one π-coCartesian morphism f: x → y' in C such that π(f) = g. In other words, π is the dual of a Grothendieck fibration.
- opposite
- The opposite category of a category is obtained by reversing the arrows. For example, if a partially ordered set is viewed as a category, taking its opposite amounts to reversing the ordering.
P
- π-accessible
- Given a cardinal number π, an object X in a category is π-accessible if
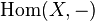 commutes with π-filtrant inductive limits.
commutes with π-filtrant inductive limits. - pointed
- A category (or ∞-category) is called pointed if it has a zero object.
- preadditive
- A category is preadditive if it is enriched over the monoidal category of abelian groups. More generally, it is R-linear if it is enriched over the monoidal category of R-modules, for R a commutative ring.
- presheaf
- Another term for a contravariant functor: a functor from a category Cop to Set is a presheaf of sets on C and a functor from Cop to sSet is a presheaf of simplicial sets or simplicial presheaf, etc. A topology on C, if any, tells which presheaf is a sheaf (with respect to that topology).
- product
- 1. The product of a family of objects Xi in a category C indexed by a set I is the projective limit
 of the functor
of the functor 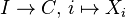 , where I is viewed as a discrete category. It is denoted by
, where I is viewed as a discrete category. It is denoted by  and is the dual of the coproduct of the family.
and is the dual of the coproduct of the family. - 2. The product of a family of categories Ci's indexed by a set I is the category denoted by
 whose class of objects is the product of the classes of objects of Ci's and whose hom-sets are
whose class of objects is the product of the classes of objects of Ci's and whose hom-sets are 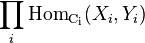 ; the morphisms are composed component-wise. It is the dual of the disjoint union.
; the morphisms are composed component-wise. It is the dual of the disjoint union. - projective
- An object A in an abelian category is projective if the functor
 is exact. It is the dual of an injective object.
is exact. It is the dual of an injective object.
Q
- Quillen
- Quillen’s theorem A provides a criterion for a functor to be a weak equivalence.
R
- reflect
- 1. A functor is said to reflect identities if it has the property: if F(k) is an identity then k is an identity as well.
- 2. A functor is said to reflect isomorphismsif it has the property: F(k) is an isomorphism then k is an isomorphism as well.
- representable
- A set-valued contravariant functor F on a category C is said to be representable if it belongs to the essential image of the Yoneda embedding
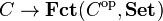 ; i.e.,
; i.e., 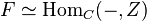 for some object Z. The object Z is said to be the representing object of F.
for some object Z. The object Z is said to be the representing object of F. - retraction
- A morphism is a retraction if it has a right inverse.
 f is a retraction of g. g is a section of f.
f is a retraction of g. g is a section of f.
S
- section
- A morphism is a section if it has a left inverse. For example, the axiom of choice says that any surjective function admits a section.
- Segal space
- Segal spaces were certain simplicial spaces, introduced as models for (∞, 1)-categories.
- simple
- An object in an abelian category is simple if it is not isomorphic to the zero object and any subobject of A is isomorphic to zero or to A.
- Simplicial localization
- Simplicial localization is a method of localizing a category.
- simplicial set
- A simplicial set is a contravariant functor from Δ to Set, where Δ is the category whose objects are the sets [n] = { 0, 1, …, n } and whose morphisms are order-preserving functions.
- skeletal
- A category is skeletal if isomorphic objects are necessarily identical.
- slice
- Given a category C and an object A in it, the slice category C/A of C over A is the category whose objects are all the morphisms in C with codomain A, whose morphisms are morphisms in C such that if f is a morphism from
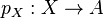 to
to 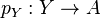 , then
, then 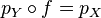 in C and whose composition is that of C.
in C and whose composition is that of C. - small
- A small category is a category in which the class of all morphisms is a set (i.e., not a proper class); otherwise large. A category is locally small if the morphisms between every pair of objects A and B form a set. Some authors assume a foundation in which the collection of all classes forms a "conglomerate", in which case a quasicategory is a category whose objects and morphisms merely form a conglomerate.[6] (NB other authors use the term "quasicategory" with a different meaning.[7]
- stable
- An ∞-category is stable if (1) it has a zero object, (2) every morphism in it admits a fiber and a cofiber and (3) a triangle in it is a fiber sequence if and only if it is a cofiber sequence.
- strict
- A morphism f in a category admitting finite limits and finite colimits is strict if the natural morphism
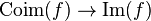 is an isomorphism.
is an isomorphism. - subcanonical
- A topology on a category is subcanonical if every representable contravariant functor on C is a sheaf with respect to that topology.[8] Generally speaking, some flat topology may fail to be subcanonical; but flat topologies appearing in practice tend to be subcanonical.
- subcategory
- A category A is a subcategory of a category B if there is an inclusion functor from A to B.
- subobject
- See subobject. For example, a subgroup is a subobject of a group.
- subquotient
- A subquotient is a quotient of a subobject.
- symmetric monoidal category
- A symmetric monoidal category is a monoidal category (i.e., a category with ⊗) that has maximally symmetric braiding.
T
- tensor category
- Usually synonymous with monoidal category (though some authors distinguish between the two concepts.)
- tensor product
- Given a monoidal category B, the tensor product of functors
 and
and 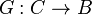 is the coend:
is the coend:
 .
.
- terminal
- 1. An object A is terminal (also called final) if there is exactly one morphism from each object to A; e.g., singletons in Set. It is the dual of an initial object.
- 2. An object A in an ∞-category C is terminal if
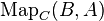 is contractible for every object B in C.
is contractible for every object B in C.
U
- universal
- 1. Given a functor
 and an object X in D, a universal morphism from X to f is an initial object in the comma category
and an object X in D, a universal morphism from X to f is an initial object in the comma category  . (Its dual is also called a universal morphism.) For example, take f to be the forgetful functor
. (Its dual is also called a universal morphism.) For example, take f to be the forgetful functor 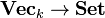 and X a set. An initial object of
and X a set. An initial object of  is a function
is a function  . That it is initial means that if
. That it is initial means that if  is another morphism, then there is a unique morphism from j to k, which consists of a linear map
is another morphism, then there is a unique morphism from j to k, which consists of a linear map 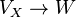 that extends k via j; that is to say,
that extends k via j; that is to say,  is the free vector space generated by X.
is the free vector space generated by X. - 2. Stated more explicitly, given f as above, a morphism
 in D is universal if and only if the natural map
in D is universal if and only if the natural map
 , then taking c to be uX one gets a universal morphism by sending the identity morphism. In other words, having a universal morphism is equivalent to the representability of the functor
, then taking c to be uX one gets a universal morphism by sending the identity morphism. In other words, having a universal morphism is equivalent to the representability of the functor 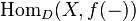 .
.
W
- Waldhausen category
- A Waldhausen category is, roughly, a category with families of cofibrations and weak equivalences.
- wellpowered
- A category is wellpowered if for each object there is only a set of pairwise non-isomorphic subobjects.
Y
- Yoneda lemma
- The Yoneda lemma says: For each set-valued contravariant functor F on C and an object X in C, there is a natural bijection
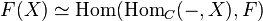 ;
;
in particular, the functor
Z
- zero
- A zero object is an object that is both initial and terminal, such as a trivial group in Grp.
Notes
- ↑ Notes on foundations: In many expositions (e.g., Vistoli), the set-theoretic issues are ignored; this means, for instance, that one does not distinguish between small and large categories and that one can arbitrarily form a localization of a category. If one believes in the existence of strongly inaccessible cardinals, then there can be a rigorous theory where statements and constructions have references to Grothendieck universes; this approach is taken, for example, in Lurie's Higher Topos Theory.
- ↑ Kashiwara–Schapira 2006, Ch. 2, Exercise 2.8.
- ↑ Mac Lane 1998, Ch. III, § 3..
- ↑ http://ncatlab.org/nlab/show/Day+convolution
- ↑ Kashiwara & Schapira 2006, exercise 8.20
- ↑ Adámek, Jiří; Herrlich, Horst; Strecker, George E (2004) [1990]. Abstract and Concrete Categories (The Joy of Cats) (PDF). New York: Wiley & Sons. p. 40. ISBN 0-471-60922-6.
- ↑ Joyal, A. (2002). "Quasi-categories and Kan complexes". Journal of Pure and Applied Algebra 175 (1-3): 207–222. doi:10.1016/S0022-4049(02)00135-4.
- ↑ Vistoli, Definition 2.57.
- ↑ Technical note: the lemma implicitly involves a choice of Set; i.e., a choice of universe.
References
- Artin, Michael; Alexandre Grothendieck, Jean-Louis Verdier, eds. (1972). Séminaire de Géométrie Algébrique du Bois Marie - 1963-64 - Théorie des topos et cohomologie étale des schémas - (SGA 4) - vol. 1. Lecture notes in mathematics (in French) 269. Berlin; New York: Springer-Verlag. xix+525. doi:10.1007/BFb0081551. ISBN 978-3-540-05896-0. Cite uses deprecated parameter
|coauthors=(help) - Kashiwara, Masaki; Schapira, Pierre (2006). "Categories and sheaves"
- Lurie, J., Higher Algebra
- Lurie, J., Higher Topos Theory
- Mac Lane, Saunders (1998). Categories for the Working Mathematician. Graduate Texts in Mathematics 5 (2nd ed.). New York, NY: Springer-Verlag. ISBN 0-387-98403-8. Zbl 0906.18001.
- Pedicchio, Maria Cristina; Tholen, Walter, eds. (2004). Categorical foundations. Special topics in order, topology, algebra, and sheaf theory. Encyclopedia of Mathematics and Its Applications 97. Cambridge: Cambridge University Press. ISBN 0-521-83414-7. Zbl 1034.18001.
- Vistoli, Angelo (2004-12-28). "Notes on Grothendieck topologies, fibered categories and descent theory". arXiv:math/0412512.
Further reading
- Groth, M., A Short Course on ∞-categories
- Cisinski's notes
- History of topos theory
- http://plato.stanford.edu/entries/category-theory/
This article is issued from Wikipedia - version of the Saturday, December 19, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.