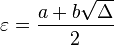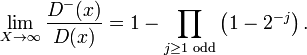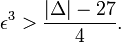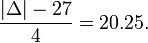Fundamental unit (number theory)

In algebraic number theory, a fundamental unit is a generator (modulo the roots of unity) for the unit group of the ring of integers of a number field, when that group has rank 1 (i.e. when the unit group modulo its torsion subgroup is infinite cyclic). Dirichlet's unit theorem shows that the unit group has rank 1 exactly when the number field is a real quadratic field, a complex cubic field, or a totally imaginary quartic field. When the unit group has rank ≥ 1, a basis of it modulo its torsion is called a fundamental system of units.^[1] Some authors use the term fundamental unit to mean any element of a fundamental system of units, not restricting to the case of rank 1 (e.g. Neukirch 1999, p. 42).

Real quadratic fields

For the real quadratic field 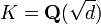$K=\mathbf{Q}(\sqrt{d})$ (with d square-free), the fundamental unit ε is commonly normalized so that $ε > 1$ (as a real number). Then it is uniquely characterized as the minimal unit among those that are greater than 1. If Δ denotes the discriminant of K, then the fundamental unit is

\varepsilon=\frac{a+b\sqrt{\Delta}}{2}

where (a, b) is the smallest solution to^[2]

x^2-\Delta y^2=\pm4

in positive integers. This equation is basically Pell's equation or the negative Pell equation and its solutions can be obtained similarly using the continued fraction expansion of 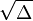$\sqrt{\Delta}$ .

Whether or not x² − Δy² = −4 has a solution determines whether or not the class group of K is the same as its narrow class group, or equivalently, whether or not there is a unit of norm −1 in K. This equation is known to have a solution if, and only if, the period of the continued fraction expansion of 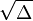$\sqrt{\Delta}$ is odd. A simpler relation can be obtained using congruences: if Δ is divisible by a prime that is congruent to 3 modulo 4, then K does not have a unit of norm −1. However, the converse does not hold as shown by the example d = 34.^[3] In the early 1990s, Peter Stevenhagen proposed a probabilistic model that led him to a conjecture on how often the converse fails. Specifically, if D(X) is the number of real quadratic fields whose discriminant Δ < X is not divisible by a prime congruent to 3 modulo 4 and D⁻(X) is those who have a unit of norm −1, then^[4]

\lim_{X\rightarrow\infty}\frac{D^-(x)}{D(x)}=1-\prod_{j\geq1\text{ odd}}\left(1-2^{-j}\right).

In other words, the converse fails about 42% of the time. As of March 2012, a recent result towards this conjecture was provided by Étienne Fouvry and Jürgen Klüners^[5] who show that the converse fails between 33% and 59% of the time.

Cubic fields

If K is a complex cubic field then it has a unique real embedding and the fundamental unit ε can be picked uniquely such that |ε| > 1 in this embedding. If the discriminant Δ of K satisfies |Δ| ≥ 33, then^[6]

\epsilon^3>\frac{|\Delta|-27}{4}.

For example, the fundamental unit of $\mathbf{Q}(\sqrt[3]{2})$ is $1+\sqrt[3]{2}+\sqrt[3]{2^2}$ whose cube is ≈ 56.9, whereas the discriminant of this field is −108 and

\frac{|\Delta|-27}{4}=20.25.

Notes

References

Alaca, Şaban; Williams, Kenneth S. (2004), Introductory algebraic number theory, Cambridge University Press, ISBN 978-0-521-54011-7
Duncan Buell (1989). Binary quadratic forms: classical theory and modern computations. Springer-Verlag. pp. 92–93. ISBN 0-387-97037-1.
Fouvry, Étienne; Klüners, Jürgen (2010), "On the negative Pell equation", Annals of Mathematics 2 (3): 2035–2104, doi:10.4007/annals.2010.172.2035, MR 2726105
Neukirch, Jürgen (1999), Algebraic Number Theory, Grundlehren der mathematischen Wissenschaften 322, Berlin: Springer-Verlag, ISBN 978-3-540-65399-8, Zbl 0956.11021, MR 1697859
Stevenhagen, Peter (1993), "The number of real quadratic fields having units of negative norm", Experimental Mathematics 2 (2): 121–136, doi:10.1080/10586458.1993.10504272, MR 1259426

External links

Weisstein, Eric W., "Fundamental Unit", MathWorld.

This article is issued from Wikipedia - version of the Friday, April 03, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.