fpqc morphism

In algebraic geometry, an fpqc morphism 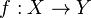$f: X \to Y$ of schemes is a faithfully flat morphism that satisfies the following equivalent conditions:

Every quasi-compact open subset of Y is the image of a quasi-compact open subset of X.
There exists a covering $V_i$ of Y by open affine subschemes such that each $V_i$ is the image of a quasi-compact open subset of X.
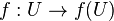Each point $x \in X$ has a neighborhood $U$ such that $f(U)$ is open and $f: U \to f(U)$ is quasi-compact.
Each point $x \in X$ has a quasi-compact neighborhood such that $f(U)$ is open affine.

Examples: An open faithfully flat morphism is fpqc.

An fpqc morphism satisfies the following properties:

The composite of fpqc morphisms is fpqc.
A base change of an fpqc morphism is fpqc.
If 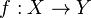$f: X \to Y$ is a morphism of schemes and if there is an open covering $V_i$ of Y such that the $f: f^{-1}(V_i) \to V_i$ is fpqc, then f is fpqc.
A faithfully flat morphism that is locally of finite presentation (i.e., fppf) is fpqc.
If 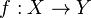$f:X \to Y$ is an fpqc morphism, a subset of Y is open in Y if and only if its inverse image under f is open in X.

Fpqc stands for the French phrase "fidèlement plat et quasi-compact", meaning "faithfully flat and quasi-compact".

References

Angelo Vistoli, "Notes on Grothendieck topologies, fibered categories and descent theory." arXiv:math.AG/0412512v4

This article is issued from Wikipedia - version of the Tuesday, April 21, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.

fpqc morphism

See also

References