Ford circle

Ford circles for q from 1 to 20. Circles with q ≤ 10 are labelled as p/q and color-coded according to q. Each circle is tangential to the base line and its neighboring circles. Irreducible fractions with the same denominator have circles of the same size.

In mathematics, a Ford circle is a circle with center at (p/q,1/(2q^{2})) and radius 1/(2q^{2}), where p/q is an irreducible fraction, i.e. p and q are coprime integers. Each Ford circle is tangent to the horizontal axis y=0, and any two circles are either tangent or disjoint from each other.[1]

History

Ford circles are a special case of mutually tangent circles; the base line can be thought of as a circle with infinite radius. Systems of mutually tangent circles were studied by Apollonius of Perga, after whom the problem of Apollonius and the Apollonian gasket are named.[2] In the 17th century René Descartes discovered Descartes' theorem, a relationship between the reciprocals of the radii of mutually tangent circles.[2]

Ford circles also appear in the Sangaku (geometrical puzzles) of Japanese mathematics. A typical problem, which is presented on an 1824 tablet in the Gunma Prefecture, covers the relationship of three touching circles with a common tangent. Given the size of the two outer large circles, what is the size of the small circle between them? The answer is equivalent to a Ford circle:[3]

{\frac {1}{\sqrt {r_{\text{middle}}}}}={\frac {1}{\sqrt {r_{\text{left}}}}}+{\frac {1}{\sqrt {r_{\text{right}}}}}.

Ford circles are named after the American mathematician Lester R. Ford, Sr., who wrote about them in 1938.[1]

Properties

The Ford circle associated with the fraction p/q is denoted by C[p/q] or C[p,q]. There is a Ford circle associated with every rational number. In addition, the line y=1 is counted as a Ford circle – it can be thought of as the Ford circle associated with infinity, which is the case p=1,q=0.

Two different Ford circles are either disjoint or tangent to one another. No two interiors of Ford circles intersect, even though there is a Ford circle tangent to the x-axis at each point on it with rational coordinates. If p/q is between 0 and 1, the Ford circles that are tangent to C[p/q] can be described variously as

  1. the circles C[r/s] where |ps-qr|=1,[1]
  2. the circles associated with the fractions r/s that are the neighbors of p/q in some Farey sequence,[1] or
  3. the circles C[r/s] where r/s is the next larger or the next smaller ancestor to p/q in the Stern–Brocot tree or where p/q is the next larger or next smaller ancestor to r/s.[1]

Ford circles can also be thought of as curves in the complex plane. The modular group of transformations of the complex plane maps Ford circles to other Ford circles.[1]

By interpreting the upper half of the complex plane as a model of the hyperbolic plane (the Poincaré half-plane model) Ford circles can also be interpreted as a tiling of the hyperbolic plane by horocycles. Any two Ford circles are congruent in hyperbolic geometry.[4] If C[p/q] and C[r/s] are tangent Ford circles, then the half-circle joining (p/q,0) and (r/s,0) that is perpendicular to the x-axis is a hyperbolic line that also passes through the point where the two circles are tangent to one another.

Ford circles are a sub-set of the circles in the Apollonian gasket generated by the lines y=0 and y=1 and the circle C[0/1].[5]

Total area of Ford circles

There is a link between the area of Ford circles, Euler's totient function 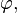\varphi , the Riemann zeta function \zeta , and Apéry's constant \zeta (3).[6] As no two Ford circles intersect, it follows immediately that the total area of the Ford circles

\left\{C[p,q]:0\leq {\frac {p}{q}}\leq 1\right\}

is less than 1. In fact the total area of these Ford circles is given by a convergent sum, which can be evaluated. From the definition, the area is

A=\sum _{q\geq 1}\sum _{(p,q)=1 \atop 1\leq p<q}\pi \left({\frac {1}{2q^{2}}}\right)^{2}.

Simplifying this expression gives

A={\frac {\pi }{4}}\sum _{q\geq 1}{\frac {1}{q^{4}}}\sum _{(p,q)=1 \atop 1\leq p<q}1={\frac {\pi }{4}}\sum _{q\geq 1}{\frac {\varphi (q)}{q^{4}}}={\frac {\pi }{4}}{\frac {\zeta (3)}{\zeta (4)}},

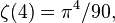where the last equality reflects the Dirichlet generating function for Euler's totient function \varphi (q). Since \zeta (4)=\pi ^{4}/90, this finally becomes

A={\frac {45}{2}}{\frac {\zeta (3)}{\pi ^{3}}}\approx 0.872284041.

References

  1. 1 2 3 4 5 6 Ford, L. R. (1938), "Fractions", The American Mathematical Monthly 45 (9): 586–601, doi:10.2307/2302799, JSTOR 2302799, MR 1524411.
  2. 1 2 Coxeter, H. S. M. (1968), "The problem of Apollonius", The American Mathematical Monthly 75: 5–15, doi:10.2307/2315097, MR 0230204.
  3. ↑ Fukagawa, Hidetosi; Pedoe, Dan (1989), Japanese temple geometry problems, Winnipeg, MB: Charles Babbage Research Centre, ISBN 0-919611-21-4, MR 1044556.
  4. ↑ Conway, John H. (1997), The sensual (quadratic) form, Carus Mathematical Monographs 26, Washington, DC: Mathematical Association of America, pp. 28–33, ISBN 0-88385-030-3, MR 1478672.
  5. ↑ Graham, Ronald L.; Lagarias, Jeffrey C.; Mallows, Colin L.; Wilks, Allan R.; Yan, Catherine H. (2003), "Apollonian circle packings: number theory", Journal of Number Theory 100 (1): 1–45, arXiv:math.NT/0009113, doi:10.1016/S0022-314X(03)00015-5, MR 1971245.
  6. ↑ Marszalek, Wieslaw (2012), "Circuits with oscillatory hierarchical Farey sequences and fractal properties", Circuits, Systems and Signal Processing 31 (4): 1279–1296, doi:10.1007/s00034-012-9392-3.

External links

This article is issued from Wikipedia - version of the Saturday, November 07, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.