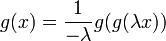Feigenbaum function

In the study of dynamical systems the term Feigenbaum function has been used to describe two different functions introduced by the physicist Mitchell Feigenbaum:

• the solution to the Feigenbaum-Cvitanović functional equation; and
• the scaling function that described the covers of the attractor of the logistic map

Functional equation

The functional equation arises in the study of one-dimensional maps that, as a function of a parameter, go through a period-doubling cascade. The functional equation is the mathematical expression of the universality of period doubling. The equation is used to specify a function g and a parameter λ by the relation

with the initial conditions

• g(0) = 1,
• g′(0) = 0, and
• g′′(0) < 0

For a particular form of solution with a quadratic dependence of the solution near x=0, the inverse 1/λ=2.5029... is one of the Feigenbaum constants.

Scaling function

The Feigenbaum scaling function provides a complete description of the attractor of the logistic map at the end of the period-doubling cascade. The attractor is a Cantor set set, and just as the middle-third Cantor set, it can be covered by a finite set of segments, all bigger than a minimal size d_n. For a fixed d_n the set of segments forms a cover Δ_n of the attractor. The ratio of segments from two consecutive covers, Δ_n and Δ_n+1 can be arranged to approximate a function σ, the Feigenbaum scaling function.

See also

• Logistic map
• Presentation function

References

• Weisstein, Eric W., "Feigenbaum Function", MathWorld.
• Feigenbaum, M. (1978). "Quanitative universality for a class of nonlinear transformations". Journal of Statistical Physics 19 (1): 25–52. Bibcode:1978JSP....19...25F. doi:10.1007/BF01020332. MR 0501179. 
• Feigenbaum, M. (1979). "The universal metric properties of non-linear transformations". Journal of Statistical Physics 21 (6): 669–706. Bibcode:1979JSP....21..669F. doi:10.1007/BF01107909. MR 0555919. 
• Feigenbaum, Mitchell J. (1980). "The transition to aperiodic behavior in turbulent systems". Communications in Mathematical Physics 77 (1): 65–86. Bibcode:1980CMaPh..77...65F. doi:10.1007/BF01205039. 
• Epstein, H.; Lascoux, J. (1981). "Analyticity properties of the Feigenbaum Function". Commun. Math. Phys. 81 (3): 437–453. Bibcode:1981CMaPh..81..437E. doi:10.1007/BF01209078. 
• Feigenbaum, Mitchell J. (1983). "Universal Behavior in Nonlinear Systems". Physica 7D: 16–39. Bibcode:1983PhyD....7...16F. doi:10.1016/0167-2789(83)90112-4.  Bound as Order in Chaos, Proceedings of the International Conference on Order and Chaos held at the Center for Nonlinear Studies, Los Alamos, New Mexico 87545,USA 24–28 May 1982, Eds. David Campbell, Harvey Rose; North-Holland Amsterdam ISBN 0-444-86727-9.
• Lanford III, Oscar E. (1982). "A computer-assisted proof of the Feigenbaum conjectures". Bull. Am. Math. Soc. 6 (3): 427–434. doi:10.1090/S0273-0979-1982-15008-X. MR 0648529. 
• Campanino, M.; Epstein, H.; Ruelle, D. (1982). "On Feigenbaums functional equation 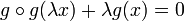". Topology 21 (2): 125–129. doi:10.1016/0040-9383(82)90001-5. MR 0641996. 
• Lanford III, Oscar E. (1984). "A shorter proof of the existence of the Feigenbaum fixed point". Commun. Math. Phys. 96 (4): 521–538. Bibcode:1984CMaPh..96..521L. doi:10.1007/BF01212533. 
• Epstein, H. (1986). "New proofs of the existence of the Feigenbaum functions". Commun. Math. Phys. 106 (3): 395–426. Bibcode:1986CMaPh.106..395E. doi:10.1007/BF01207254. 
• Eckmann, Jean-Pierre; Wittwer, Peter (1987). "A complete proof of the Feigenbaum Conjectures". J. Stat. Phys. 46 (3/4): 455. Bibcode:1987JSP....46..455E. doi:10.1007/BF01013368. MR 0883539. 
• Stephenson, John; Wang, Yong (1991). "Relationships between the solutions of Feigenbaum's equation". Appl. Math. Lett. 4 (3): 37–39. doi:10.1016/0893-9659(91)90031-P. MR 1101871. 
• Stephenson, John; Wang, Yong (1991). "Relationships between eigenfunctions associated with solutions of Feigenbaum's equation". Appl. Math. Lett. 4 (3): 53–56;. doi:10.1016/0893-9659(91)90035-T. MR 1101875. 
• Briggs, Keith (1991). "A precise calculation of the Feigenbaum constants". Math. Comp. 57 (195): 435–439. Bibcode:1991MaCom..57..435B. doi:10.1090/S0025-5718-1991-1079009-6. MR 1079009. 
• Tsygvintsev, Alexei V.; Mestel, Ben D.; Obaldestin, Andrew H. (2002). "Continued fractions and solutions of the Feigenbaum-Cvitanović equation". C. R. Acad. Sci. Paris, Ser. I 334 (8): 683–688. doi:10.1016/S1631-073X(02)02330-0. 
• Mathar, Richard J. (2010). "Chebyshev series representation of Feigenbaum's period-doubling function". arXiv:1008.4608 [math.DS]. 
• Varin, V. P. (2011). "Spectral properties of the period-doubling operator". KIAM Preprint 9.