Excluded point topology

In mathematics, the excluded point topology is a topology where exclusion of a particular point defines openness. Formally, let X be any set and p ∈ X. The collection

T = {S ⊆ X: p ∉ S or S = X;}

of subsets of X is then the excluded point topology on X. There are a variety of cases which are individually named:

• If X has two points we call it the Sierpiński space. This case is somewhat special and is handled separately.
• If X is finite (with at least 3 points) we call the topology on X the finite excluded point topology
• If X is countably infinite we call the topology on X the countable excluded point topology
• If X is uncountable we call the topology on X the uncountable excluded point topology

A generalization / related topology is the open extension topology. That is if 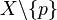has the discrete topology then the open extension topology will be the excluded point topology.

This topology is used to provide interesting examples and counterexamples. Excluded point topology is also connected and that is clear since the only open set containing the excluded point is X itself and hence X cannot be written as disjoint union of two proper open subsets.

See also

• Sierpiński space
• Particular point topology
• Alexandrov topology
• Finite topological space
• Fort space

References

• Steen, Lynn Arthur; Seebach, J. Arthur Jr. (1995) [1978], Counterexamples in Topology (Dover reprint of 1978 ed.), Berlin, New York: Springer-Verlag, ISBN 978-0-486-68735-3, MR 507446 

my notes Taha el Turki.[1]