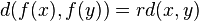Dilation (metric space)
In mathematics, a dilation is a function  from a metric space into itself that satisfies the identity
from a metric space into itself that satisfies the identity
for all points 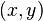 , where
, where  is the distance from
is the distance from  to
to 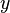 and
and  is some positive real number.[1]
is some positive real number.[1]
In Euclidean space, such a dilation is a similarity of the space.[2] Dilations change the size but not the shape of an object or figure.
Every dilation of a Euclidean space that is not a congruence has a unique fixed point[3] that is called the center of dilation.[4] Some congruences have fixed points and others do not.[5]
See also
References
- ↑ Montgomery, Richard (2002), A tour of subriemannian geometries, their geodesics and applications, Mathematical Surveys and Monographs 91, American Mathematical Society, Providence, RI, p. 122, ISBN 0-8218-1391-9, MR 1867362.
- ↑ King, James R. (1997), "An eye for similarity transformations", in King, James R.; Schattschneider, Doris, Geometry Turned On: Dynamic Software in Learning, Teaching, and Research, Mathematical Association of America Notes 41, Cambridge University Press, pp. 109–120, ISBN 9780883850992. See in particular p. 110.
- ↑ Audin, Michele (2003), Geometry, Universitext, Springer, Proposition 3.5, pp. 80–81, ISBN 9783540434986.
- ↑ Gorini, Catherine A. (2009), The Facts on File Geometry Handbook, Infobase Publishing, p. 49, ISBN 9781438109572.
- ↑ Carstensen, Celine; Fine, Benjamin; Rosenberger, Gerhard (2011), Abstract Algebra: Applications to Galois Theory, Algebraic Geometry and Cryptography, Walter de Gruyter, p. 140, ISBN 9783110250091.
This article is issued from Wikipedia - version of the Tuesday, January 06, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.