Dehn surgery

In topology, a branch of mathematics, a Dehn surgery, named after Max Dehn, is a construction used to modify 3-manifolds. The process takes as input a 3-manifold together with a link. Dehn surgery can be thought of as a two-stage process: drilling and Dehn filling.

Definitions

• Given a 3-manifold and a link , drilled along is obtained by removing an open tubular neighborhood of from . drilled along is also known as the link complement, since if one removed the corresponding closed tubular neighborhood from one obtains a manifold diffeomorphic to .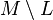

• Given a 3-manifold with torus boundary components, we may glue in a solid torus by a homeomorphism (resp. diffeomorphism) of its boundary to the torus boundary component of the original 3-manifold. There are many inequivalent ways of doing this, in general. This process is called Dehn filling.

• Dehn surgery on a 3-manifold containing a link consists of drilling out a tubular neighbourhood of the link together with Dehn filling on all the components of the boundary corresponding to the link.

We can pick two oriented simple closed curves m and ℓ on the boundary torus of the 3-manifold that generate the fundamental group of the torus. This gives any simple closed curve 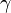on that torus two coordinates p and q, each coordinate corresponding to the algebraic intersection of the curve with m and ℓ respectively. These coordinates only depend on the homotopy class of .

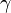We can specify a homeomorphism of the boundary of a solid torus to T by having the meridian curve of the solid torus map to a curve homotopic to 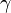. As long as the meridian maps to the surgery slope , the resulting Dehn surgery will yield a 3-manifold that will not depend on the specific gluing (up to homeomorphism). The ratio p/q is called the surgery coefficient.

In the case of links in the 3-sphere or more generally an oriented homology sphere, there is a canonical choice of the meridians and longitudes of T. The longitude is chosen so that it is null-homologous in the knot complement—equivalently, if it is the boundary of a Seifert surface. The meridian is the curve that bounds a disc in the tubular neighbourhood of the link. When the ratios p/q are all integers, the surgery is called an integral surgery. Such surgeries are closely related to handlebodies, cobordism and Morse functions.

Results

Every closed, orientable, connected 3-manifold is obtained by performing Dehn surgery on a link in the 3-sphere. This result, the Lickorish-Wallace theorem, was first proven by Wallace in 1960 and independently by Lickorish in a stronger form in 1962. Via the now well-known relation between genuine surgery and cobordism, this result is equivalent to the theorem that the oriented cobordism group of 3-manifolds is trivial, originally due to Vladimir Abramovich Rokhlin in 1951.

Since orientable 3-manifolds can all be generated by suitably decorated links, one might ask how distinct surgery presentations of a given 3-manifold might be related. The answer is called the Kirby calculus.

See also

• Hyperbolic Dehn surgery
• Tubular neighborhood
• Surgery on manifolds, in the general sense, also called spherical modification.

References

• Dehn, Max (1938), "Die Gruppe der Abbildungsklassen", Acta Mathematica 69 (1): 135–206, doi:10.1007/BF02547712 .
• Thom, René (1954), "Quelques propriétés globales des variétés différentiables", Commentarii Mathematici Helvetici 28: 17–86, doi:10.1007/BF02566923, MR 0061823 
• Kirby, Rob (1978), "A calculus for framed links in S³", Inventiones Mathematicae 45 (1): 35–56, doi:10.1007/BF01406222, MR 467753 .
• Fenn, Roger; Rourke, Colin (1979), "On Kirby's calculus of links", Topology 18 (1): 1–15, doi:10.1016/0040-9383(79)90010-7, MR 0528232 .
• Gompf, Robert; Stipsicz, András (1999), 4-Manifolds and Kirby Calculus, Graduate Studies in Mathematics 20, Providence, RI: American Mathematical Society, doi:10.1090/gsm/020, ISBN 0-8218-0994-6, MR 1707327 .