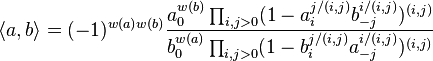Contou-Carrère symbol

In mathematics, the Contou-Carrère symbol 〈a,b〉 is a Steinberg symbol defined on pairs of invertible elements of the ring of Laurent power series over an Artinian ring k, taking values in the group of units of k. It was introduced by Contou-Carrère (1994).

Definition

If k is an Artinian local ring, then any invertible formal Laurent series a with coefficients in k can be written uniquely as

a=a_0t^{w(a)}\prod_{i\ne 0}(1-a_it^i)

where w(a) is an integer, the elements a_i are in k, and are in m if i is negative, and is a unit if i = 0.

The Contou-Carrère symbol 〈a,b〉 of a and b is defined to be

\langle a,b\rangle=(-1)^{w(a)w(b)}\frac{a_0^{w(b)}\prod_{i,j>0}(1-a_i^{j/(i,j)}b_{-j}^{i/(i,j)})^{(i,j)}} {b_0^{w(a)}\prod_{i,j>0}(1-b_i^{j/(i,j)}a_{-j}^{i/(i,j)})^{(i,j)}}

References

Contou-Carrère, Carlos (1994), "Jacobienne locale, groupe de bivecteurs de Witt universel, et symbole modéré", C. R. Acad. Sci. Paris Sér. I Math. (in French) 318 (8): 743–746, MR 1272340

This article is issued from Wikipedia - version of the Wednesday, February 25, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.