Compound of ten tetrahedra

Compound of ten tetrahedra
Type	regular compound
Coxeter symbol	2{5,3}[10{3,3}]2{3,5}[1]
Index	UC6, W25
Elements (As a compound)	10 tetrahedra: F = 40, E = 60, V = 20
Dual compound	Self-dual
Symmetry group	icosahedral (Ih)
Subgroup restricting to one constituent	chiral tetrahedral (T)

The compound of ten tetrahedra is one of the five regular polyhedral compounds. This polyhedron can be seen as either a stellation of the icosahedron or a compound. This compound was first described by Edmund Hess in 1876.

It can be seen as a faceting of a regular dodecahedron.

As a compound

As a spherical tiling

It can also be seen as the compound of ten tetrahedra with full icosahedral symmetry (I_h). It is one of five regular compounds constructed from identical Platonic solids.

It shares the same vertex arrangement as a dodecahedron.

The compound of five tetrahedra represents two chiral halves of this compound.

It can be made from the compound of five cubes by replacing each cube with a stella octangula on the cube's vertices.

As a stellation

This polyhedron is a stellation of the icosahedron, and given as Wenninger model index 25.

Stellation diagram	Stellation core	Convex hull
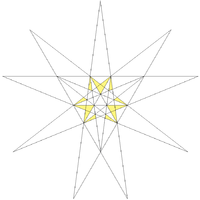	Icosahedron	Dodecahedron

As a facetting

Ten tetrahedra in a dodecahedron.

It is also a facetting of the dodecahedron, as shown at left.

As a simple polyhedron

If it is treated as a simple non-convex polyhedron without self-intersecting surfaces, it has 180 faces (120 triangles and 60 concave quadrilaterals), 122 vertices (60 with degree 3, 30 with degree 4, 12 with degree 5, and 20 with degree 12), and 300 edges, giving an Euler characteristic of 122-300+180 = +2.

See also

• Compound of five tetrahedra

References

• Wenninger, Magnus (1974). Polyhedron Models. Cambridge University Press. ISBN 0-521-09859-9. 
• Coxeter, Harold Scott MacDonald; Du Val, P.; Flather, H. T.; Petrie, J. F. (1999). The fifty-nine icosahedra (3rd ed.). Tarquin. ISBN 978-1-899618-32-3. MR 676126.  (1st Edn University of Toronto (1938))
• H.S.M. Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8, 3.6 The five regular compounds, pp.47-50, 6.2 Stellating the Platonic solids, pp.96-104

External links

• Weisstein, Eric W., "Tetrahedron 10-Compound", MathWorld.
• VRML model:
• Compounds of 5 and 10 Tetrahedra by Sándor Kabai, The Wolfram Demonstrations Project.
• Richard Klitzing, 3D compound,

Notable stellations of the icosahedron
Regular	Uniform duals			Regular compounds			Regular star	Others
(Convex) icosahedron	Small triambic icosahedron	Medial triambic icosahedron	Great triambic icosahedron	Compound of five octahedra	Compound of five tetrahedra	Compound of ten tetrahedra	Great icosahedron	Excavated dodecahedron	Final stellation
The stellation process on the icosahedron creates a number of related polyhedra and compounds with icosahedral symmetry.