Complex analytic space

In mathematics, a complex analytic space is a generalization of a complex manifold which allows the presence of singularities. Complex analytic spaces are locally ringed spaces which are locally isomorphic to local model spaces, where a local model space is an open subset of the vanishing locus of a finite set of holomorphic functions.

Definition

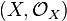Denote the constant sheaf on a topological space with value $\mathbb{C}$ by $\underline{\mathbb{C}}$ . A $\mathbb{C}$ -space is a locally ringed space $(X, \mathcal{O}_X)$ whose structure sheaf is an algebra over $\underline{\mathbb{C}}$ .

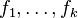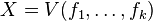Choose an open subset $U$ of some complex affine space $\mathbb{C}^n$ , and fix finitely many holomorphic functions $f_1,\dots,f_k$ in $U$ . Let $X=V(f_1,\dots,f_k)$ be the common vanishing locus of these holomorphic functions, that is, 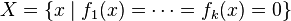$X=\{x\mid f_1(x)=\cdots=f_k(x)=0\}$ . Define a sheaf of rings on $X$ by letting $\mathcal{O}_X$ be the restriction to $X$ of $\mathcal{O}_U/(f_1, \ldots, f_k)$ , where $\mathcal{O}_U$ is the sheaf of holomorphic functions on $U$ . 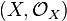Then the locally ringed $\mathbb{C}$ -space $(X, \mathcal{O}_X)$ is a local model space.

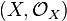A complex analytic space is a locally ringed $\mathbb{C}$ -space $(X, \mathcal{O}_X)$ which is locally isomorphic to a local model space.

Morphisms of complex analytic spaces are defined to be morphisms of the underlying locally ringed spaces, they are also called holomorphic maps.

References

Grauert and Remmert, Complex Analytic Spaces
Grauert, Peternell, and Remmert, Encyclopaedia of Mathematical Sciences 74: Several Complex Variables VII

This article is issued from Wikipedia - version of the Wednesday, July 29, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.

Complex analytic space

Definition

See also

References