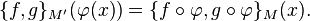Poisson manifold
A Poisson structure on a smooth manifold  is a Lie bracket
is a Lie bracket 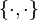 (called a Poisson bracket in this special case) on the algebra
(called a Poisson bracket in this special case) on the algebra  of smooth functions on
of smooth functions on  , subject to the Leibniz Rule
, subject to the Leibniz Rule
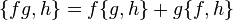 .
.
Said in another manner, it is a Lie-algebra structure on the vector space of smooth functions on  such that
such that 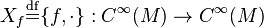 is a vector field for each smooth function
is a vector field for each smooth function  , which we call the Hamiltonian vector field associated to
, which we call the Hamiltonian vector field associated to  . These vector fields span a completely integrable singular foliation, each of whose maximal integral sub-manifolds inherits a symplectic structure. One may thus informally view a Poisson structure on a smooth manifold as a smooth partition of the ambient manifold into even-dimensional symplectic leaves, which are not necessarily of the same dimension.
. These vector fields span a completely integrable singular foliation, each of whose maximal integral sub-manifolds inherits a symplectic structure. One may thus informally view a Poisson structure on a smooth manifold as a smooth partition of the ambient manifold into even-dimensional symplectic leaves, which are not necessarily of the same dimension.
Poisson structures are one instance of Jacobi structures, introduced by André Lichnerowicz in 1977.[1] They were further studied in the classical paper of Alan Weinstein,[2] where many basic structure theorems were first proved, and which exerted a huge influence on the development of Poisson geometry — which today is deeply entangled with non-commutative geometry, integrable systems, topological field theories and representation theory, to name a few.
Definition
Let  be a smooth manifold. Let
be a smooth manifold. Let  denote the real algebra of smooth real-valued functions on
denote the real algebra of smooth real-valued functions on  , where multiplication is defined pointwise. A Poisson bracket (or Poisson structure) on
, where multiplication is defined pointwise. A Poisson bracket (or Poisson structure) on  is an
is an 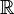 -bilinear map
-bilinear map
satisfying the following three conditions:
- Skew symmetry:
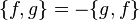 .
. - Jacobi identity:
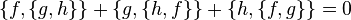 .
. - Leibniz's Rule:
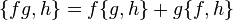 .
.
The first two conditions ensure that 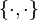 defines a Lie-algebra structure on
defines a Lie-algebra structure on  , while the third guarantees that for each
, while the third guarantees that for each 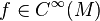 , the adjoint
, the adjoint  is a derivation of the commutative product on
is a derivation of the commutative product on  , i.e., is a vector field
, i.e., is a vector field 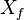 . It follows that the bracket
. It follows that the bracket 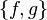 of functions
of functions  and
and  is of the form
is of the form 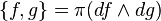 , where
, where  is a smooth bi-vector field.
is a smooth bi-vector field.
Conversely, given any smooth bi-vector field 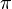 on
on  , the formula
, the formula 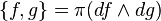 defines a bilinear skew-symmetric bracket
defines a bilinear skew-symmetric bracket 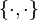 that automatically obeys Leibniz's rule. The condition that the ensuing
that automatically obeys Leibniz's rule. The condition that the ensuing 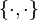 be a Poisson bracket — i.e., satisfy the Jacobi identity — can be characterized by the non-linear partial differential equation
be a Poisson bracket — i.e., satisfy the Jacobi identity — can be characterized by the non-linear partial differential equation ![[\pi,\pi] = 0](../I/m/4c968956574aefcf785f5920b467253d.png) , where
, where
denotes the Schouten–Nijenhuis bracket on multi-vector fields. It is customary and convenient to switch between the bracket and the bi-vector points of view, and we shall do so below.
Symplectic Leaves
A Poisson manifold is naturally partitioned into regularly immersed symplectic manifolds, called its symplectic leaves.
Note that a bi-vector field can be regarded as a skew homomorphism 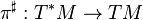 . The rank of
. The rank of 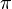 at a point
at a point  is then the rank of the induced linear mapping
is then the rank of the induced linear mapping 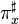 . Its image consists of the values
. Its image consists of the values 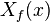 of all Hamiltonian vector fields evaluated at
of all Hamiltonian vector fields evaluated at  . A point
. A point  is called regular for a Poisson structure
is called regular for a Poisson structure 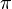 on
on  if and only if the rank of
if and only if the rank of 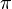 is constant on an open neighborhood of
is constant on an open neighborhood of  ; otherwise, it is called a singular point. Regular points form an open dense subspace
; otherwise, it is called a singular point. Regular points form an open dense subspace 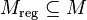 ; when
; when 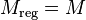 , we call the Poisson structure itself regular.
, we call the Poisson structure itself regular.
An integral sub-manifold for the (singular) distribution 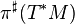 is a path-connected sub-manifold
is a path-connected sub-manifold 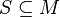 satisfying
satisfying 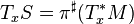 for all
for all 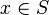 . Integral sub-manifolds of
. Integral sub-manifolds of 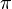 are automatically regularly immersed manifolds, and maximal integral sub-manifolds of
are automatically regularly immersed manifolds, and maximal integral sub-manifolds of 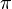 are called the leaves of
are called the leaves of 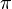 . Each leaf
. Each leaf 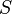 carries a natural symplectic form
carries a natural symplectic form 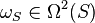 determined by the condition
determined by the condition  = - \{ f,g \}(x)](../I/m/8d5b6251d864e505e0b90b92668ccfa5.png) for all
for all 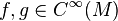 and
and 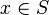 . Correspondingly, one speaks of the symplectic leaves of
. Correspondingly, one speaks of the symplectic leaves of 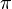 .[3] Moreover, both the space
.[3] Moreover, both the space 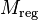 of regular points and its complement are saturated by symplectic leaves, so symplectic leaves may be either regular or singular.
of regular points and its complement are saturated by symplectic leaves, so symplectic leaves may be either regular or singular.
Examples
- Every manifold
 carries the trivial Poisson structure
carries the trivial Poisson structure 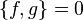 .
. - Every symplectic manifold
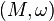 is Poisson, with the Poisson bi-vector
is Poisson, with the Poisson bi-vector 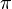 equal to the inverse
equal to the inverse 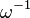 of the symplectic form
of the symplectic form  .
. - The dual
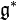 of a Lie algebra
of a Lie algebra ![(\mathfrak{g},[\cdot,\cdot])](../I/m/f1201e3ed9b1b1e8b2ed6655b2b35f99.png) is a Poisson manifold. A coordinate-free description can be given as follows:
is a Poisson manifold. A coordinate-free description can be given as follows: 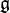 naturally sits inside
naturally sits inside  , and the rule
, and the rule ![\{ X,Y \} \stackrel{\text{df}}{=} [X,Y]](../I/m/f592c18311fdd5cd2c92d981db6b7d1b.png) for each
for each 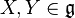 induces a linear Poisson structure on
induces a linear Poisson structure on 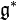 , i.e., one for which the bracket of linear functions is again linear. Conversely, any linear Poisson structure must be of this form.
, i.e., one for which the bracket of linear functions is again linear. Conversely, any linear Poisson structure must be of this form. - Let
 be a (regular) foliation of dimension
be a (regular) foliation of dimension  on
on  and
and 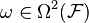 a closed foliation two-form for which
a closed foliation two-form for which 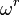 is nowhere-vanishing. This uniquely determines a regular Poisson structure on
is nowhere-vanishing. This uniquely determines a regular Poisson structure on  by requiring that the symplectic leaves of
by requiring that the symplectic leaves of 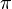 be the leaves
be the leaves 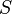 of
of  equipped with the induced symplectic form
equipped with the induced symplectic form  .
.
Poisson Maps
If 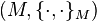 and
and 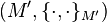 are two Poisson manifolds, then a smooth mapping
are two Poisson manifolds, then a smooth mapping 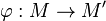 is called a Poisson map if it respects the Poisson structures, namely, if for all
is called a Poisson map if it respects the Poisson structures, namely, if for all  and smooth functions
and smooth functions 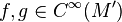 , we have:
, we have:
In terms of Poisson bi-vectors, the condition that a map be Poisson is tantamount to requiring that 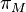 and
and 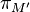 be
be  -related.
-related.
Poisson manifolds are the objects of a category  , with Poisson maps as morphisms.
, with Poisson maps as morphisms.
Examples of Poisson maps:
- The Cartesian product
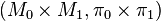 of two Poisson manifolds
of two Poisson manifolds 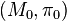 and
and 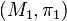 is again a Poisson manifold, and the canonical projections
is again a Poisson manifold, and the canonical projections 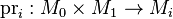 , for
, for 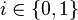 , are Poisson maps.
, are Poisson maps. - The inclusion mapping of a symplectic leaf, or of an open subspace, is a Poisson map.
It must be highlighted that the notion of a Poisson map is fundamentally different from that of a symplectic map. For instance, with their standard symplectic structures, there do not exist Poisson maps 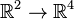 , whereas symplectic maps abound.
, whereas symplectic maps abound.
One interesting, and somewhat surprising, fact is that any Poisson manifold is the codomain/image of a surjective, submersive Poisson map from a symplectic manifold. [4][5][6]
See also
Notes
- ↑ Lichnerowicz, A. (1977). "Les variétés de Poisson et leurs algèbres de Lie associées". J. Diff. Geom. 12 (2): 253–300. MR 0501133.
- ↑ Weinstein, A. (1983). "The local structure of Poisson manifolds". J. Diff. Geom. 18 (3): 523–557.
- ↑ Fernandes, R.L.; Marcut, I. (2014). Lectures on Poisson Geometry. Yet unpublished lecture notes.
- ↑ Crainic, M.; Marcut, I. (2011). "On the existence of symplectic realizations". J. Symplectic Geom. 9 (4): 435–444.
- ↑ Karasev, M. (1987). "Analogues of objects of Lie group theory for nonlinear Poisson brackets". Math.USSR Izv. 28: 497–527.
- ↑ Weinstein, A. (1983). "The local structure of Poisson manifolds". J. Diff. Geom. 18 (3): 523–557.
References
- Bhaskara, K. H.; Viswanath, K. (1988). Poisson algebras and Poisson manifolds. Longman. ISBN 0-582-01989-3.
- Cannas da Silva, A.; Weinstein, A. (1999). Geometric models for noncommutative algebras. AMS Berkeley Mathematics Lecture Notes, 10.
- Crainic, M.; Fernandes, R.L. (2004). "Integrability of Poisson Brackets". J. Diff. Geom. 66 (1): 71–137.
- Crainic, M.; Marcut, I. (2011). "On the existence of symplectic realizations". J. Symplectic Geom. 9 (4): 435–444.
- Dufour, J.-P.; Zung, N.T. (2005). Poisson Structures and Their Normal Forms 242. Birkhäuser Progress in Mathematics.
- Fernandes, R.L.; Marcut, I. (2014). Lectures on Poisson Geometry. Yet unpublished lecture notes.
- Guillemin, V.; Sternberg, S. (1984). Symplectic Techniques in Physics. New York: Cambridge Univ. Press. ISBN 0-521-24866-3.
- Karasev, M. (1987). "Analogues of objects of Lie group theory for nonlinear Poisson brackets". Math.USSR Izv. 28: 497–527.
- Kirillov, A. A. (1976). "Local Lie algebras". Russ. Math. Surv. 31 (4): 55–75. doi:10.1070/RM1976v031n04ABEH001556.
- Libermann, P.; Marle, C.-M. (1987). Symplectic geometry and analytical mechanics. Dordrecht: Reidel. ISBN 90-277-2438-5.
- Lichnerowicz, A. (1977). "Les variétés de Poisson et leurs algèbres de Lie associées". J. Diff. Geom. 12 (2): 253–300. MR 0501133.
- Marcut, I. (2013). Normal forms in Poisson geometry. PhD Thesis: Utrecht University. Available at thesis
- Vaisman, I. (1994). Lectures on the Geometry of Poisson Manifolds. Birkhäuser. See also the review by Ping Xu in the Bulletin of the AMS.
- Weinstein, A. (1983). "The local structure of Poisson manifolds". J. Diff. Geom. 18 (3): 523–557.
- Weinstein, A. (1998). "Poisson geometry". Differential Geometry and its Applications 9 (1-2): 213–238.
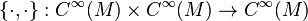
![[\cdot,\cdot]: {\mathfrak{X}^{p}}(M) \times {\mathfrak{X}^{q}}(M) \to {\mathfrak{X}^{p + q - 1}}(M)](../I/m/9b4e8cfac33ba9578bef45c7abd077b0.png)