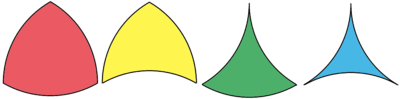
Circular triangle


Convex circular triangle
Circular horn triangle

In geometry, a circular triangle is a triangle with circular arc edges.

Construction

Special cases

Reuleaux triangle
Arbelos

A convex circular triangle may be constructed by three circles intersecting each other and represents the area of intersection. Its edges are all curved outwards. The sum of the internal angles of a circular triangle are greater than 180°. A reuleaux triangle is a special case as an equilateral triangle where the centers of each arcs are on the opposite vertex.

A circular horn triangle is a similar concept, but represents the area interior to 3 mutually tangent circles so all of the internal angles are zero.[1] The arbelos is a special case with three collinear vertices and three semicircular edges.[2]

Other circular triangles can have a mixture of convex and concave circular arc edges.

Long arcs can produce concave figures regulars of whether individual edges are curved inwards or outwards. Inward curved arcs can create self-intersecting forms, such as the a triquetra figure:

Tessellations

Circular tessellation containing lenses and concave circular triangle faces

Circular triangles can be seen in tessellation.

See also

References

  1. ↑ The Geometry of the Circular Horn Triangle Edward Kasner and Aida Kalish National Mathematics Magazine Vol. 18, No. 8 (May, 1944), pp. 299–304
  2. ↑ Boas, Harold P. (2006), "Reflections on the arbelos" (PDF), American Mathematical Monthly 113 (3): 236–249, doi:10.2307/27641891, MR 2204487.

External links

Wikimedia Commons has media related to Circular triangle.
This article is issued from Wikipedia - version of the Thursday, December 24, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.