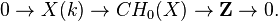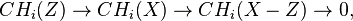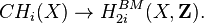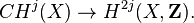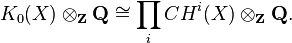Chow ring
In algebraic geometry, the Chow ring (named after W. L. Chow) of a smooth algebraic variety over any field is an algebro-geometric analog of the cohomology ring of a complex variety considered as a topological space. The elements of the Chow ring are formed out of actual subvarieties (so-called algebraic cycles), and the multiplicative structure is derived from the intersection of subvarieties. The Chow groups carry rich information about an algebraic variety, and they are correspondingly hard to compute in general.
Rational equivalence and Chow groups
For what follows, define a variety over a field k to be an integral scheme of finite type over k. For any scheme X of finite type over k, an algebraic cycle on X means a finite linear combination of subvarieties of X with integer coefficients. (Here and below, subvarieties are understood to be closed in X, unless stated otherwise.) For a natural number i, the group Zi(X) of i-dimensional cycles (or i-cycles, for short) on X is the free abelian group on the set of i-dimensional subvarieties of X.
For a variety W of dimension i + 1 and any rational function f on W which is not identically zero, the divisor of f is the i-cycle
where the sum runs over all i-dimensional subvarieties Z of W and the integer ordZ(f) denotes the order of vanishing of f along Z. (Thus ordZ(f) is negative if f has a pole along Z.) The definition of the order of vanishing requires some care for W singular.[1]
For a scheme X of finite type over k, the group of i-cycles rationally equivalent to zero is the subgroup of Zi(X) generated by the cycles (f) for all (i+1)-dimensional subvarieties W of X and all nonzero rational functions f on W. The Chow group CHi(X) of i-dimensional cycles on X is the quotient group of Zi(X) by the subgroup of cycles rationally equivalent to zero. Sometimes one writes [Z] for the class of a subvariety Z in the Chow group.
For example, when X is a variety of dimension n, the Chow group CHn-1(X) is the divisor class group of X. When X is smooth over k, this is isomorphic to the Picard group of line bundles on X.
The Chow ring
When the scheme X is smooth over a field k, the Chow groups form a ring, not just a graded abelian group. Namely, when X is smooth over k, define CHi(X) to be the Chow group of codimension-i cycles on X. (When X is a variety of dimension n, this just means that CHi(X) = CHn-i(X).) Then the groups CH*(X) form a commutative graded ring, with a product:
The product arises from intersecting algebraic cycles. For example, if Y and Z are smooth subvarieties of X of codimension i and j respectively, and if Y and Z intersect transversely, then the product [Y][Z] in CHi+j(X) is the sum of the irreducible components of the intersection Y ∩ Z, which all have codimension i+j.
More generally, in various cases, intersection theory constructs an explicit cycle that represents the product [Y][Z] in the Chow ring. For example, if Y and Z are subvarieties of complementary dimension (meaning that their dimensions sum to the dimension of X) whose intersection has dimension zero, then [Y][Z] is equal to the sum of the points of the intersection with coefficients called intersection numbers. For any subvarieties Y and Z of a smooth scheme X over k, with no assumption on the dimension of the intersection, Fulton–MacPherson's intersection theory constructs a canonical element of the Chow groups of Y ∩ Z whose image in the Chow groups of X is the product [Y][Z].[2]
Examples
The Chow ring of projective space Pn over any field k is the ring
where H is the class of any hyperplane (the zero locus of a single linear function). Furthermore, any subvariety Y of degree a and codimension r in projective space is rationally equivalent to aHr. It follows that for any two subvarieties Y and Z of complementary dimension in Pn and degrees a, b respectively, their product in the Chow ring is simply
where Hn is the class of a k-rational point in Pn. For example, if Y and Z intersect transversely, it follows that Y ∩ Z is a zero-cycle of degree ab. If the base field k is algebraically closed, this means that there are exactly ab points of intersection; this is a version of Bézout's theorem, a classic result of enumerative geometry.
For other algebraic varieties, Chow groups can have richer behavior. For example, let X be an elliptic curve over a field k. Then the Chow group of zero-cycles on X fits into an exact sequence
Thus the Chow group of an elliptic curve X is closely related to the group X(k) of k-rational points of X. When k is a number field, X(k) is called the Mordell–Weil group of X, and some of the deepest problems in number theory are attempts to understand this group. When k is the complex numbers, the example of an elliptic curve shows that Chow groups can be uncountable abelian groups.
Functoriality
For a proper morphism f: X → Y of schemes over k, there is a pushforward homomorphism f*: CHi(X) → CHi(Y) for each integer i. For example, for a proper scheme X over k, this gives a homomorphism CH0(X) → Z, which takes a closed point in X to its degree over k. (A closed point in X has the form Spec(E) for a finite extension field E of k, and its degree means the degree of the field E over k.)
For a flat morphism f: X → Y of schemes over k with fibers of dimension r (possibly empty), there is a pullback homomorphism f*: CHi(Y) → CHi+r(X).
A key computational tool for Chow groups is the localization sequence, as follows. For a scheme X over a field k and a closed subscheme Z of k, there is an exact sequence
where the first homomorphism is the pushforward associated to the proper morphism Z → X, and the second homomorphism is pullback with respect to the flat morphism X − Z → X.[3] The localization sequence can be extended to the left using a generalization of Chow groups, (Borel–Moore) motivic homology groups, also known as higher Chow groups.[4]
For any morphism f: X → Y of smooth schemes over k, there is a pullback homomorphism f*: CHi(Y) → CHi(X), which is in fact a ring homomorphism CH*(Y) → CH*(X).
Cycle maps
There are several homomorphisms (known as cycle maps) from Chow groups to more computable theories.
First, for a scheme X over the complex numbers, there is a homomorphism from Chow groups to Borel–Moore homology:[5]
The factor of 2 appears because an i-dimensional subvariety of X has real dimension 2i. When X is smooth over the complex numbers, this cycle map can be rewritten using Poincaré duality as a homomorphism
In this case (X smooth over C), these homomorphisms form a ring homomorphism from the Chow ring to the cohomology ring. Intuitively, this is because the products in both the Chow ring and the cohomology ring describe the intersection of cycles.
For a smooth complex projective variety, the cycle map from the Chow ring to ordinary cohomology factors through a richer theory, Deligne cohomology.[6] This incorporates the Abel–Jacobi map from cycles homologically equivalent to zero to the intermediate Jacobian. The exponential sequence shows that CH1(X) maps isomorphically to Deligne cohomology, but that fails for CHj(X) with j > 1.
For a scheme X over an arbitrary field k, there is an analogous cycle map from Chow groups to (Borel–Moore) etale homology. When X is smooth over k, this homomorphism can be identified with a ring homomorphism from the Chow ring to etale cohomology.[7]
Relation to K-theory
An (algebraic) vector bundle E on a smooth scheme X over a field has Chern classes ci(E) in CHi(X), with the same formal properties as in topology.[8] The Chern classes give a close connection between vector bundles and Chow groups. Namely, let K0(X) be the Grothendieck group of vector bundles on X. As part of the Grothendieck–Riemann–Roch theorem, Grothendieck showed that the Chern character gives an isomorphism
This isomorphism shows the importance of rational equivalence, compared to any other adequate equivalence relation on algebraic cycles.
Conjectures
Some of the deepest conjectures in algebraic geometry and number theory are attempts to understand Chow groups. For example:
- The Mordell–Weil theorem implies that the divisor class group CHn-1(X) is finitely generated for any variety X of dimension n over a number field. It is an open problem whether all Chow groups are finitely generated for every variety over a number field. The Bloch–Kato conjecture on values of L-functions predicts that these groups are finitely generated. Moreover, the rank of the group of cycles modulo homological equivalence, and also of the group of cycles homologically equivalent to zero, should be equal to the order of vanishing of an L-function of the given variety at certain integer points. Finiteness of these ranks would also follow from the Bass conjecture in algebraic K-theory.
- For a smooth complex projective variety X, the Hodge conjecture predicts the image (tensored with the rationals Q) of the cycle map from the Chow groups to singular cohomology. For a smooth projective variety over a finitely generated field (such as a finite field or number field), the Tate conjecture predicts the image (tensored with Ql) of the cycle map from Chow groups to l-adic cohomology.
- For a smooth projective variety X over any field, the Bloch–Beilinson conjecture predicts a filtration on the Chow groups of X (tensored with the rationals) with strong properties.[9] The conjecture would imply a tight connection between the singular or etale cohomology of X and the Chow groups of X.
- For example, let X be a smooth complex projective surface. The Chow group of zero-cycles on X maps onto the integers by the degree homomorphism; let K be the kernel. If the geometric genus h0(X, Ω2) is not zero, Mumford showed that K is "infinite-dimensional" (not the image of any finite-dimensional family of zero-cycles on X).[10] The Bloch–Beilinson conjecture would imply a satisfying converse, Bloch's conjecture on zero-cycles: for a smooth complex projective surface X with geometric genus zero, K should be finite-dimensional; more precisely, it should map isomorphically to the group of complex points of the Albanese variety of X.[11]
Variants
Fulton and MacPherson extended the Chow ring to singular varieties by defining the "operational Chow ring" and more generally a bivariant theory associated to any morphism of schemes.[12] This is in a sense the most elementary extension of the Chow ring to singular varieties; other theories such as motivic cohomology map to the operational Chow ring.
Arithmetic Chow groups are an amalgamation of Chow groups of varieties over Q together with a component encoding Arakelov-theoretical information, that is, differential forms on the associated complex manifold.
History
Rational equivalence of divisors (known as linear equivalence) was studied in various forms during the 19th century, leading to the ideal class group in number theory and the Jacobian variety in the theory of algebraic curves. For higher-codimension cycles, rational equivalence was introduced by Severi in the 1930s. In 1956, Wei-Liang Chow gave an influential proof that the intersection product is well-defined on cycles modulo rational equivalence for a smooth quasi-projective variety, using Chow's moving lemma. Starting in the 1970s, Fulton and MacPherson gave the current standard foundation for Chow groups, working with singular varieties wherever possible. In their theory, the intersection product for smooth varieties is constructed by deformation to the normal cone.[13]
References
- ↑ Fulton. Intersection Theory, section 1.2 and Appendix A.3.
- ↑ Fulton, Intersection Theory, section 8.1.
- ↑ Fulton, Intersection Theory, Proposition 1.8.
- ↑ Bloch, Algebraic cycles and higher K-groups; Voevodsky, Triangulated categories of motives over a field, section 2.2 and Proposition 4.2.9.
- ↑ Fulton, Intersection Theory, section 19.1
- ↑ Voisin, Hodge Theory and Complex Algebraic Geometry, v. 1, section 12.3.3; v. 2, Theorem 9.24.
- ↑ Deligne, Cohomologie Etale (SGA 4 1/2), Expose 4.
- ↑ Fulton, Intersection Theory, section 3.2 and Example 8.3.3.
- ↑ Voisin, Hodge Theory and Complex Algebraic Geometry, v. 2, Conjecture 11.21.
- ↑ Voisin, Hodge Theory and Complex Algebraic Geometry, v. 2, Theorem 10.1.
- ↑ Voisin, Hodge Theory and Complex Algebraic Geometry, v. 2, Ch. 11.
- ↑ Fulton, Intersection Theory, Chapter 17.
- ↑ Fulton, Intersection Theory, Chapters 5, 6, 8.
- Bloch, Spencer (1986), "Algebraic cycles and higher K-theory", Advances in Mathematics 61 (3): 267–304, doi:10.1016/0001-8708(86)90081-2, ISSN 0001-8708, MR 0852815
- Chow, Wei-Liang (1956), "On equivalence classes of cycles in an algebraic variety", Annals of Mathematics 64: 450–479, doi:10.2307/1969596, ISSN 0003-486X, MR 0082173
- Deligne, Pierre (1977), Cohomologie Etale (SGA 4 1/2), Springer-Verlag, ISBN 978-3-540-08066-4, MR 0463174
- Fulton, William (1998), Intersection Theory, Berlin, New York: Springer-Verlag, ISBN 978-0-387-98549-7, MR 1644323
- Severi, Francesco (1932), "La serie canonica e la teoria delle serie principali di gruppi di punti sopra una superficie algebrica", Commentarii Mathematici Helvetici 4: 268–326, JFM 58.1229.01
- Voevodsky, Vladimir (2000), "Triangulated categories of motives over a field", Cycles, Transfers, and Motivic Homology Theories, Princeton University Press, pp. 188–238, ISBN 9781400837120, MR 1764202
- Voisin, Claire (2002), Hodge Theory and Complex Algebraic Geometry (2 vols.), Cambridge University Press, ISBN 978-0-521-71801-1, MR 1997577
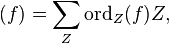
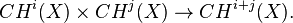
![CH^*(\mathbf{P}^n) \cong \mathbb{Z}[H]/(H^{n + 1}),](../I/m/f595de78f425c9f3deeddc8b68a565ca.png)
![[Y] \cdot [Z] = a\, b\, H^n](../I/m/54d83667db169518c3837810a4a0e657.png)