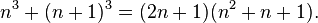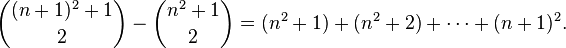Centered cube number

35 points in a body-centered cubic lattice, forming two cubical layers around a central point

A centered cube number is a centered figurate number that counts the number of points in a three-dimensional pattern formed by a point surrounded by concentric cubical layers of points, with i2 points on the square faces of the ith layer. Equivalently, it is the number of points in a body-centered cubic pattern within a cube that has n + 1 points along each of its edges.

The first few centered cube numbers are

1, 9, 35, 91, 189, 341, 559, 855, 1241, 1729, 2331, 3059, 3925, 4941, 6119, 7471, 9009, ... (sequence A005898 in OEIS).

Formulas

The centered cube number for a pattern with n concentric layers around the central point is given by the formula[1]

n^3 + (n + 1)^3 = (2n+1)(n^2+n+1).

The same number can also be expressed as a trapezoidal number (difference of two triangular numbers), or a sum of consecutive numbers, as[2]

\binom{(n+1)^2+1}{2}-\binom{n^2+1}{2} = (n^2+1)+(n^2+2)+\cdots+(n+1)^2.

Properties

Because of the factorization (2n+1)(n^2+n+1), it is impossible for a centered cube number to be a prime number.[3] The only centered cube number that is also a square number is 9.[4][5]

See also

References

  1. ↑ Deza, Elena; Deza, Michel (2012), Figurate Numbers, World Scientific, pp. 121–123, ISBN 9789814355483.
  2. ↑ Lanski, Charles (2005), Concepts in Abstract Algebra, American Mathematical Society, p. 22, ISBN 9780821874288.
  3. ↑ "Sloane's A005898 ", The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
  4. ↑ Stroeker, R. J. (1995), "On the sum of consecutive cubes being a perfect square", Compositio Mathematica 97 (1–2): 295–307, MR 1355130.
  5. ↑ O'Shea, Owen; Dudley, Underwood (2007), The Magic Numbers of the Professor, MAA Spectrum, Mathematical Association of America, p. 17, ISBN 9780883855577.

External links

This article is issued from Wikipedia - version of the Sunday, January 18, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.