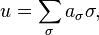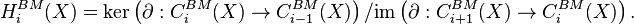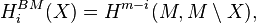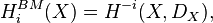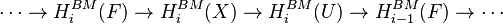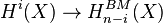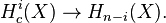Borel–Moore homology

In topology, Borel−Moore homology or homology with closed support is a homology theory for locally compact spaces, introduced by Borel and Moore (1960).

For reasonable compact spaces, Borel−Moore homology coincides with the usual singular homology. For non-compact spaces, each theory has its own advantages. In particular, a closed oriented submanifold defines a class in Borel–Moore homology, but not in ordinary homology unless the submanifold is compact.

Note: The equivariant cohomology theory for spaces with an action of a group G is sometimes called Borel cohomology; it is defined as H*_G(X) = H*((EG × X)/G). That is not related to the subject of this article.

Definition

There are several ways to define Borel−Moore homology. They all coincide for reasonable spaces such as manifolds and CW complexes.

Definition via sheaf cohomology

For any locally compact space X, Borel-Moore homology with integral coefficients is defined as the cohomology of the dual of the chain complex which computes sheaf cohomology with compact support.^[1] As a result, there is a short exact sequence analogous to the universal coefficient theorem: $0 \to \text{Ext}^1_{\mathbf Z}(H^{i+1}_c(X,\mathbf Z),\mathbf Z) \to H_i^{BM}(X,\mathbf Z) \to \text{Hom}(H^i_c(X,\mathbf Z),\mathbf Z) \to 0.$

In what follows, the coefficients Z are not written.

Definition via locally finite chains

The singular homology of a topological space X is defined as the homology of the chain complex of singular chains, that is, finite linear combinations of continuous maps from the simplex to X. The Borel−Moore homology of a reasonable locally compact space X, on the other hand, is isomorphic to the homology of the chain complex of locally finite singular chains. "Reasonable" means here that X is locally contractible, σ-compact, and of finite dimension.^[2]

In more detail, let C_i^BM(X) be the abelian group of formal (infinite) sums

u = \sum_{\sigma} a_{\sigma } \sigma,

where σ runs over the set of all continuous maps from the standard i-simplex Δⁱ to X and each a_σ is an integer, such that for each compact subset S of X, only finitely many maps σ whose image meets S have nonzero coefficient in u. Then the usual definition of the boundary ∂ of a singular chain makes these abelian groups into a chain complex:

\cdots \to C_2^{BM}(X) \to C_1^{BM}(X) \to C_0^{BM}(X) \to 0.

The Borel−Moore homology groups H_i^BM(X) are the homology groups of this chain complex. That is,

H^{BM}_i (X) = \ker \left (\partial : C_i^{BM}(X) \to C_{i-1}^{BM}(X) \right )/ \text{im} \left (\partial :C_{i+1}^{BM}(X) \to C_i^{BM}(X) \right ).

If X is compact, then every locally finite chain is in fact finite. So, given that X is "reasonable" in the sense above, Borel−Moore homology H_i^BM(X) coincides with the usual singular homology H_i(X) for X compact.

Definition via compactifications

Suppose that X is homeomorphic to the complement of a closed subcomplex S in a finite CW complex Y. Then Borel–Moore homology H_i^BM(X) is isomorphic to the relative homology H_i(Y, S). Under the same assumption on X, the one-point compactification of X is homeomorphic to a finite CW complex. As a result, Borel–Moore homology can be viewed as the relative homology of the one-point compactification with respect to the added point.

Definition via Poincaré duality

Let X be any locally compact space with a closed embedding into an oriented manifold M of dimension m. Then

H^{BM}_i(X)= H^{m-i}(M,M\setminus X),

where in the right hand side, the usual relative cohomology is meant.^[3]

Definition via the dualizing complex

For any locally compact space X of finite dimension, let $D X$ be the dualizing complex of $X$ . Then

H^{BM}_i (X)=H^{-i} (X, D_X),

where in the right hand side, hypercohomology is meant.^[4]

Example

Let X = R² − 0, the plane minus the origin. The usual homology of X is Z in degrees 0 and 1 (and zero otherwise), while the Borel−Moore homology of X is Z in degrees 1 and 2 (and zero otherwise). In this example, the natural homomorphism H_i(X) → H_i^BM(X) is zero for all integers i. For example, the usual homology H₁(X) is generated by a circle around the origin, but this maps to zero in Borel–Moore homology, because a finite 1-chain representing the circle is the boundary of a locally finite 2-chain whose image is the region inside the circle. The Borel–Moore homology H₁^BM(X) is generated by a locally finite 1-chain whose image is (for example) the ray from 0 to ∞ in the real line R inside R².

Properties

Borel−Moore homology is a covariant functor with respect to proper maps. That is, a proper map f: X → Y induces a pushforward homomorphism H_i^BM(X) → H_i^BM(Y) for all integers i. In contrast to ordinary homology, there is no pushforward on Borel−Moore homology for an arbitrary continuous map f. As a counterexample, one can consider the non-proper inclusion R² − 0 → R².

Borel−Moore homology is a contravariant functor with respect to inclusions of open subsets. That is, for U open in X, there is a natural pullback or restriction homomorphism H_i^BM(X) → H_i^BM(U).

For any locally compact space X and any closed subset F, with U = X − F the complement, there is a long exact localization sequence:^[5]

\cdots \to H^{BM}_i (F) \to H^{BM}_i (X) \to H^{BM}_i (U) \to H^{BM}_{i-1} (F) \to \cdots

Borel−Moore homology is homotopy invariant in the sense that for any space X, there is an isomorphism H_i^BM(X) → H_i+1^BM(X × R). The shift in dimension means that Borel−Moore homology is not homotopy invariant in the naive sense. For example, the Borel−Moore homology of Euclidean space Rⁿ is isomorphic to Z in degree n and is otherwise zero.

Poincaré duality extends to non-compact manifolds using Borel–Moore homology. Namely, for an oriented n-manifold X, Poincaré duality is an isomorphism

H^i(X) \to H_{n-i}^{BM}(X)

for all integers i. A different version of Poincaré duality for non-compact manifolds is the isomorphism from cohomology with compact support to usual homology:

H^i_c(X) \to H_{n-i}(X).

A key advantage of Borel−Moore homology is that every oriented manifold M of dimension n (in particular, every smooth complex algebraic variety), not necessarily compact, has a fundamental class [M] ∈ H_n^BM(M). This is just the sum of all top dimensional simplices in a specific triangulation. In fact, in Borel−Moore homology, one can define a fundamental class for arbitrary (possibly singular) complex varieties. In this case the set of smooth points $M reg \subset M$ has complement of (real) codimension at least 2, and by the long exact sequence above the top dimensional homologies of $M$ and $M reg$ are canonically isomorphic. The fundamental class of $M$ is then defined to be the fundamental class of $M reg$ .^[6]

Notes

↑ B. Iversen. Cohomology of sheaves. Section IX.1.
↑ G. Bredon. Sheaf theory. Corollary V.12.21.
↑ B. Iversen. Cohomology of sheaves. Theorem IX.4.7.
↑ B. Iversen. Cohomology of sheaves. Equation IX.4.1.
↑ B. Iversen. Cohomology of sheaves. Equation IX.2.1.
↑ W. Fulton. Intersection theory. Lemma 19.1.1.

References

Borel, Armand; Moore, John C. (1960), "Homology theory for locally compact spaces", The Michigan Mathematical Journal 7: 137–159, doi:10.1307/mmj/1028998385, ISSN 0026-2285, MR 0131271
Bredon, Glen E. (1997), Sheaf theory (2nd ed.), Berlin, New York: Springer-Verlag, ISBN 978-0-387-94905-5, MR 1481706
Fulton, William (1998), Intersection theory (2nd ed.), Berlin, New York: Springer-Verlag, ISBN 978-3-540-62046-4, MR 1644323
Iversen, Birger (1986), Cohomology of sheaves, Berlin: Springer-Verlag, ISBN 3-540-16389-1, MR 0842190

This article is issued from Wikipedia - version of the Wednesday, December 30, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.