Pointed set

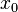In mathematics, a pointed set[1][2] (also based set[1] or rooted set[3]) is an ordered pair (X, x_0) where X is a set and x_0 is an element of X called the base point,[2] also spelled basepoint.[4]:10–11

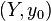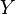Maps between pointed sets (X, x_0) and (Y, y_0) (called based maps,[5] pointed maps,[4] or point-preserving maps[6]) are functions from X to Y that map one basepoint to another, i.e. a map 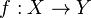f : X \to Y such that f(x_0) = y_0. This is usually denoted

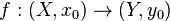f : (X, x_0) \to (Y, y_0).

Pointed sets may be regarded as a rather simple algebraic structure. In the sense of universal algebra, they are structures with a single nullary operation which picks out the basepoint.[7]

The class of all pointed sets together with the class of all based maps form a category. In this category the pointed singleton set 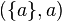(\{a\}, a) is an initial object and a terminal object,[1] i.e. a zero object.[4]:226 There is a faithful functor from usual sets to pointed sets, but it is not full and these categories are not equivalent.[8]:44 In particular, the empty set is not a pointed set, for it has no element that can be chosen as base point.[9]

The category of pointed sets and based maps is equivalent to but not isomorphic with the category of sets and partial functions.[6] One textbook notes that "This formal completion of sets and partial maps by adding “improper,” “infinite” elements was reinvented many times, in particular, in topology (one-point compactification) and in theoretical computer science."[10]

The category of pointed sets and pointed maps is isomorphic to the co-slice category \mathbf{1} \downarrow \mathbf{Set}, where \mathbf{1} is a singleton set.[8]:46[11]

The category of pointed sets and pointed maps has both products and co-products, but it is not a distributive category.[9]

Many algebraic structures are pointed sets in a rather trivial way. For example, groups are pointed sets by choosing the identity element as the basepoint, so that group homomorphisms are point-preserving maps.[12]:24 This observation can be restated in category theoretic terms as the existence of a forgetful functor from groups to pointed sets.[12]:582

A pointed set may be seen as a pointed space under the discrete topology or as a vector space over the field with one element.[13]

As "rooted set" the notion naturally appears in the study of antimatroids[3] and transportation polytopes.[14]

See also

References

  1. 1 2 3 Mac Lane (1998) p.26
  2. 1 2 Grégory Berhuy (2010). An Introduction to Galois Cohomology and Its Applications. London Mathematical Society Lecture Note Series 377. Cambridge University Press. p. 34. ISBN 0-521-73866-0. Zbl 1207.12003.
  3. 1 2 Korte, Bernhard; Lovász, László; Schrader, Rainer (1991), Greedoids, Algorithms and Combinatorics 4, New York, Berlin: Springer-Verlag, chapter 3, ISBN 3-540-18190-3, Zbl 0733.05023
  4. 1 2 3 Joseph Rotman (2008). An Introduction to Homological Algebra (2nd ed.). Springer Science & Business Media. ISBN 978-0-387-68324-9.
  5. ↑ Maunder, C. R. F. (1996), Algebraic Topology, Dover, p. 31.
  6. 1 2 Lutz Schröder (2001). "Categories: a free tour". In Jürgen Koslowski and Austin Melton. Categorical Perspectives. Springer Science & Business Media. p. 10. ISBN 978-0-8176-4186-3.
  7. ↑ Saunders Mac Lane; Garrett Birkhoff (1999) [1988]. Algebra (3rd ed.). American Mathematical Soc. p. 497. ISBN 978-0-8218-1646-2.
  8. 1 2 J. Adamek, H. Herrlich, G. Stecker, (18th January 2005) Abstract and Concrete Categories-The Joy of Cats
  9. 1 2 F. W. Lawvere; Stephen Hoel Schanuel (2009). Conceptual Mathematics: A First Introduction to Categories (2nd ed.). Cambridge University Press. pp. 296–298. ISBN 978-0-521-89485-2.
  10. ↑ Neal Koblitz; B. Zilber; Yu. I. Manin (2009). A Course in Mathematical Logic for Mathematicians. Springer Science & Business Media. p. 290. ISBN 978-1-4419-0615-1.
  11. ↑ Francis Borceux; Dominique Bourn (2004). Mal'cev, Protomodular, Homological and Semi-Abelian Categories. Springer Science & Business Media. p. 131. ISBN 978-1-4020-1961-6.
  12. 1 2 Paolo Aluffi (2009). Algebra: Chapter 0. American Mathematical Soc. ISBN 978-0-8218-4781-7.
  13. ↑ Haran, M. J. Shai (2007), "Non-additive geometry" (PDF), Compositio Mathematica 143 (3): 618–688, MR 2330442. On p. 622, Haran writes "We consider \mathbb{F}-vector spaces as finite sets X with a distinguished ‘zero’ element..."
  14. ↑ Klee, V.; Witzgall, C. (1970) [1968]. "Facets and vertices of transportation polytopes". In George Bernard Dantzig. Mathematics of the Decision Sciences. Part 1. American Mathematical Soc. ISBN 0-8218-1111-0. OCLC 859802521.

    External links

    This article is issued from Wikipedia - version of the Tuesday, April 21, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.