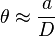Angular distance
In mathematics (in particular geometry and trigonometry) and all natural sciences (including astronomy, geophysics, etc.), the angular distance (angular separation, apparent distance, or apparent separation) between two point objects, as observed from a location different from either of these objects, is the size of the angle between the two directions originating from the observer and pointing towards these two objects.
Use
Angular distance (or separation) is technically synonymous to angle itself, but is meant to suggest the (often large or unknown) linear distance between these objects (for instance stars, as they are observed from Earth).
Measurement
Since the angular distance (or separation) is conceptually identical to an angle, it is measured in the same units, such as degrees or radians, using instruments such as goniometers or optical instruments specially designed to point in well-defined directions and record the corresponding angles (such as telescopes).
Equation
In order to calculate the angular distance 
in arcseconds for binary star systems, extrasolar planets, solar system objects and other astronomical objects, we use orbital distance (semi-major axis), 
, in AU divided by stellar distance 
in parsecs, per the small-angle approximation for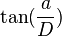 
:
See also
- Angular mil
- Gradian
- Hour angle
- Central angle
- Angular diameter
- Angular displacement
- Great-circle distance