Absolutely convex set
A set C in a real or complex vector space is said to be absolutely convex if it is convex and balanced.
Properties
A set  is absolutely convex if and only if for any points
is absolutely convex if and only if for any points 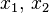 in
in  and any numbers
and any numbers 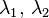 satisfying
satisfying
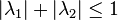 the sum
the sum  belongs to
belongs to  .
.
Since the intersection of any collection of absolutely convex sets is absolutely convex then for any subset A of a vector space one can define its absolutely convex hull to be the intersection of all absolutely convex sets containing A.
Absolutely convex hull

The light gray area is the Absolutely convex hull of the cross.
The absolutely convex hull of the set A assumes the following representation
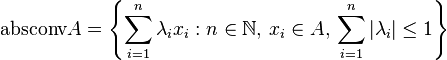 .
.
See also
| The Wikibook Algebra has a page on the topic of: Vector spaces |
- vector (geometric), for vectors in physics
- Vector field
References
- Robertson, A.P.; W.J. Robertson (1964). Topological vector spaces. Cambridge Tracts in Mathematics 53. Cambridge University Press. pp. 4–6.
| ||||||||||||||||||||||||||||||||||
This article is issued from Wikipedia - version of the Tuesday, July 21, 2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.