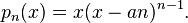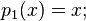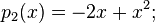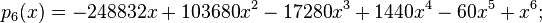Abel polynomials

The Abel polynomials in mathematics form a polynomial sequence, the nth term of which is of the form

p_n(x)=x(x-an)^{n-1}. \,

The sequence is named after Niels Henrik Abel (1802-1829), the Norwegian mathematician.

This polynomial sequence is of binomial type: conversely, every polynomial sequence of binomial type may be obtained from the Abel sequence in the umbral calculus.

Examples

For $a=1$ , the polynomials are (sequence A137452 in OEIS)

p_0(x)=1;

p_1(x)=x;

p_2(x)=-2x+x^2;

p_3(x)=9x-6x^2+x^3;

p_4(x)=-64x +48x^2-12x^3+x^4;

For $a=2$ , the polynomials are

p_0(x)=1;

p_1(x)=x;

p_2(x)=-4x+x^2;

p_3(x)=36x-12x^2+x^3;

p_4(x)=-512x +192x^2-24x^3+x^4;

p_5(x)=10000x-4000x^2+600x^3-40x^4+x^5;

p_6(x)=-248832x+103680x^2-17280x^3+1440x^4-60x^5+x^6;

References

Rota, Gian-Carlo; Shen, Jianhong; Taylor, Brian D. (1997). "All Polynomials of Binomial Type Are Represented by Abel Polynomials". Annali della Scuola Normale Superiore di Pisa - Classe di Scienze Sér. 4 25 (3–4): 731–738. MR 1655539. Zbl 1003.05011.

External links

Weisstein, Eric W., "Abel Polynomial", MathWorld.

This article is issued from Wikipedia - version of the Sunday, August 31, 2014. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.