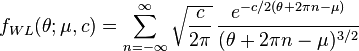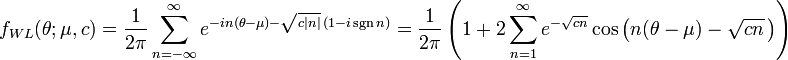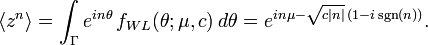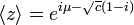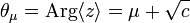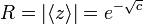Wrapped Lévy distribution
In probability theory and directional statistics, a wrapped Lévy distribution is a wrapped probability distribution that results from the "wrapping" of the Lévy distribution around the unit circle.
Description
The pdf of the wrapped Lévy distribution is
where the value of the summand is taken to be zero when 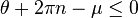 ,
,  is the scale factor and
is the scale factor and 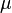 is the location parameter. Expressing the above pdf in terms of the characteristic function of the Lévy distribution yields:
is the location parameter. Expressing the above pdf in terms of the characteristic function of the Lévy distribution yields:
In terms of the circular variable  the circular moments of the wrapped Lévy distribution are the characteristic function of the Lévy distribution evaluated at integer arguments:
the circular moments of the wrapped Lévy distribution are the characteristic function of the Lévy distribution evaluated at integer arguments:
where  is some interval of length
is some interval of length  . The first moment is then the expectation value of z, also known as the mean resultant, or mean resultant vector:
. The first moment is then the expectation value of z, also known as the mean resultant, or mean resultant vector:
The mean angle is
and the length of the mean resultant is
See also
References
- Fisher, N. I. (1996). Statistical Analysis of Circular Data. Cambridge University Press. ISBN 978-0-521-56890-6. Retrieved 2010-02-09.