Whitehead's lemma (Lie algebras)
In algebra, Whitehead's lemma on a Lie algebra representation is an important step toward the proof of Weyl's theorem on complete reducibility. Let 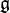 be a semisimple Lie algebra over a field of characteristic zero, V a finite-dimensional module over it and
be a semisimple Lie algebra over a field of characteristic zero, V a finite-dimensional module over it and  a linear map such that
a linear map such that ![f([x, y]) = xf(y) - yf(x)](../I/m/8dd918a6d76c193de3139681fd41e77a.png) . The lemma states that there exists a vector v in V such that
. The lemma states that there exists a vector v in V such that 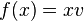 for all x.
for all x.
The lemma may be interpreted in terms of Lie algebra cohomology. The proof of the lemma uses a Casimir element.
References
- Jacobson, Nathan, Lie algebras, Republication of the 1962 original. Dover Publications, Inc., New York, 1979. ISBN 0-486-63832-4