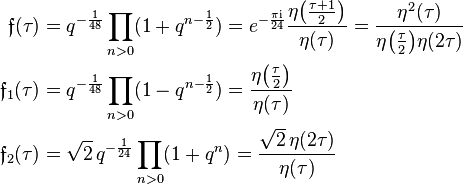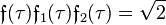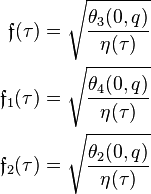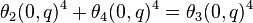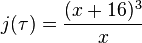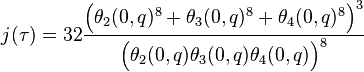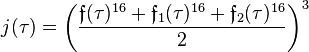Weber modular function

In mathematics, the Weber modular functions are a family of three modular functions f, f1, and f2, studied by Heinrich Martin Weber.

Definition

Let 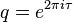q = e^{2\pi i \tau} where τ is an element of the upper half-plane.

\begin{align} \mathfrak{f}(\tau) &= q^{-\frac{1}{48}}\prod_{n>0}(1+q^{n-\frac{1}{2}}) = e^{-\frac{\pi\rm{i}}{24}}\frac{\eta\big(\frac{\tau+1}{2}\big)}{\eta(\tau)}=\frac{\eta^2(\tau)}{\eta\big(\tfrac{\tau}{2}\big)\eta(2\tau)}\\ \mathfrak{f}_1(\tau) &= q^{-\frac{1}{48}}\prod_{n>0}(1-q^{n-\frac{1}{2}}) = \frac{\eta\big(\tfrac{\tau}{2}\big)}{\eta(\tau)}\\ \mathfrak{f}_2(\tau) &= \sqrt2\, q^{-\frac{1}{24}}\prod_{n>0}(1+q^{n})= \frac{\sqrt2\,\eta(2\tau)}{\eta(\tau)} \end{align}

where\eta(\tau) is the Dedekind eta function. Note the eta quotients immediately imply that,

\mathfrak{f}(\tau)\mathfrak{f}_1(\tau)\mathfrak{f}_2(\tau) =\sqrt{2}

The transformation τ → –1/τ fixes f and exchanges f1 and f2. So the 3-dimensional complex vector space with basis f, f1 and f2 is acted on by the group SL2(Z).

Relation to theta functions

Let the argument of the Jacobi theta function be the nome 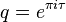q = e^{\pi i \tau}. Then,

\begin{align} \mathfrak{f}(\tau) &= \sqrt{\frac{\theta_3(0,q)}{\eta(\tau)}} \\ \mathfrak{f}_1(\tau) &= \sqrt{\frac{\theta_4(0,q)}{\eta(\tau)}} \\ \mathfrak{f}_2(\tau) &= \sqrt{\frac{\theta_2(0,q)}{\eta(\tau)}} \\ \end{align}

Thus,

\mathfrak{f}_1(\tau)^8+\mathfrak{f}_2(\tau)^8 = \mathfrak{f}(\tau)^8

which is simply a consequence of the well known identity,

\theta_2(0,q)^4+\theta_4(0,q)^4 = \theta_3(0,q)^4

Relation to j-function

The three roots of the cubic equation,

j(\tau)=\frac{(x+16)^3}{x}

where j(τ) is the j-function are given by 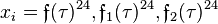x_i = \mathfrak{f}(\tau)^{24}, \mathfrak{f}_1(\tau)^{24}, \mathfrak{f}_2(\tau)^{24}. Also, since,

j(\tau)=32\frac{\Big(\theta_2(0,q)^8+\theta_3(0,q)^8+\theta_4(0,q)^8\Big)^3}{\Big(\theta_2(0,q)\theta_3(0,q)\theta_4(0,q)\Big)^8}

then,

j(\tau)=\left(\frac{\mathfrak{f}(\tau)^{16}+\mathfrak{f}_1(\tau)^{16}+\mathfrak{f}_2(\tau)^{16}}{2}\right)^3

See also

References