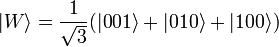W state
The W state is an entangled quantum state of three qubits which has the following shape
and which is remarkable for representing a specific type of multipartite entanglement and for occurring in several applications in quantum information theory.
Properties
The W state is the representative of one of the two non-biseparable[1] classes of three-qubit states (the other being the GHZ state) which cannot be transformed (not even probabilistically) into each other by local quantum operations.[2] Thus 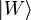 and
and 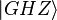 represent two very different kinds of tripartite entanglement.
represent two very different kinds of tripartite entanglement.
This difference is, for example, illustrated by the following interesting property of the W state: if one of the three qubits is lost, the state of the remaining 2-qubit system is still entangled. This robustness of W-type entanglement contrasts strongly with the Greenberger-Horne-Zeilinger state which is fully separable after loss of one qubit.
The states in the W class can be distinguished from all other three-qubit states by means of multipartite entanglement measures. In particular, W states have non-zero entanglement across any bipartition[3] while the 3-tangle vanishes, which is also non-zero for GHZ-type states.[2]
Generalization
The notion of W state has been generalized for 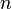 qubits[2] and then refers to the quantum superpostion with equal expansion coefficients of all possible pure states in which exactly one of the qubits in an "excited state"
qubits[2] and then refers to the quantum superpostion with equal expansion coefficients of all possible pure states in which exactly one of the qubits in an "excited state" 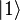 , while all other ones are in the "ground state"
, while all other ones are in the "ground state" 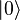
Both the robustness against particle loss and the LOCC-inequivalence with the (generalized) GHZ state also hold for the 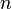 -qubit W state.
-qubit W state.
Applications
In systems in which a single qubit is stored in an ensemble of many two level systems the logical "1" is often represented by the W state while the logical "0" is represented by the state 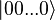 . Here the W state's robustness against particle loss is a very beneficial property ensuring good storage properties of these ensemble based quantum memories.[4]
. Here the W state's robustness against particle loss is a very beneficial property ensuring good storage properties of these ensemble based quantum memories.[4]
References
- ↑ A pure state
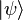 of
of 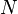 parties is called biseparable, if one can find a partition of the parties in two disjoint subsets
parties is called biseparable, if one can find a partition of the parties in two disjoint subsets 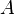 and
and 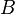 with
with 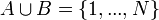 such that
such that 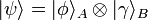 , i.e.
, i.e. 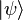 is a product state with respect to he partition
is a product state with respect to he partition 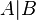 .
. - ↑ 2.0 2.1 2.2 W. Dür, G. Vidal, and J.I. Cirac (2000). "Three qubits can be entangled in two inequivalent ways". Phys. Rev. A 62: 062314. arXiv:quant-ph/0005115. Bibcode:2000PhRvA..62f2314D. doi:10.1103/PhysRevA.62.062314.
- ↑ A bipartition of the three qubits
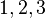 is any grouping
is any grouping 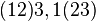 and
and 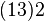 in which two qubits are considered to belong to the same party. The three qubit state can then be considered as a state on
in which two qubits are considered to belong to the same party. The three qubit state can then be considered as a state on 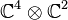 and studied with bipartite entanglement measures.
and studied with bipartite entanglement measures. - ↑ M. Fleischhauer and M. D. Lukin (2002). "Quantum memory for photons: Dark-state polaritons" 65. p. 022314. arXiv:quant-ph/0106066. Bibcode:2002PhRvA..65b2314F. doi:10.1103/PhysRevA.65.022314.