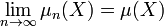Vitali–Hahn–Saks theorem
In mathematics, the Vitali–Hahn–Saks theorem, introduced by Vitali (1907), Hahn (1922), and Saks (1933), states that given μn for each integer n >0, a countably additive function defined on a fixed sigma-algebra Σ, with values in a given Banach space B, such that
exists for every set X in Σ, then μ is also countably additive. In other words, the limit of a sequence of vector measures is a vector measure.
References
- Hahn, H. (1922), "Über Folgen linearer Operationen.", Monath. f. Math. (in German) 32: 3–88, doi:10.1007/bf01696876
- Saks, Stanislaw (1933), "Addition to the Note on Some Functionals", Transactions of the American Mathematical Society (Providence, R.I.: American Mathematical Society) 35 (4): 965–970, doi:10.2307/1989603, ISSN 0002-9947
- Vitali, G. (1907), "Sull' integrazione per serie.", Rendiconti del Circolo Matematico di Palermo (in Italian) 23: 137–155, doi:10.1007/BF03013514