Vecten points

Vecten points

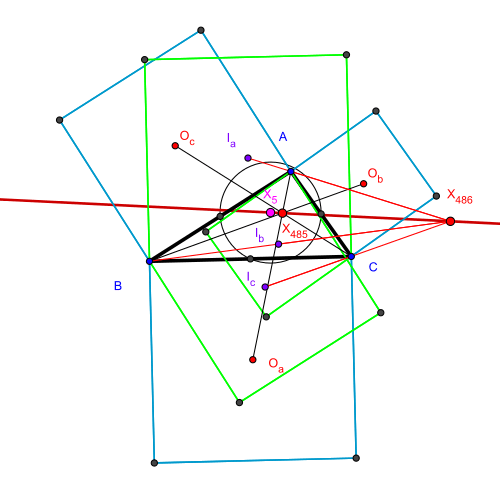
Outer Vecten point

Let ABC be any given plane triangle. On the sides BC, CA, AB of the triangle, construct outwardly drawn three squares with centres 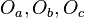respectively. Then the lines 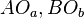and 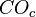are concurrent. The point of concurrence outer is Vecten point of the triangle ABC.

In Clark Kimberling's Encyclopedia of Triangle Centers, the outer Vecten point is denoted by X(485).^[1] The Vecten points are named after an early 19th-century French mathematician named Vecten, who taught mathematics with Gergonne in Nîmes and published a study of the figure of three squares on the sides of a triangle in 1817.^[2]

Inner Vecten point

Let ABC be any given plane triangle. On the sides BC, CA, AB of the triangle, construct inwardly drawn three squares respectively with centres 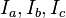respectively. Then the lines 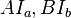and 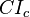are concurrent. The point of concurrence is inner Vecten point of the triangle ABC.

In Clark Kimberling's Encyclopedia of Triangle Centers, the inner Vecten point is denoted by X(486).^[1]

The line 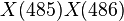meets the Euler line at the Nine point center of the triangle . The Vecten points lie on the Kiepert hyperbola

See also

• Napoleon points, a pair of triangle centers constructed in an analogous way using equilateral triangles instead of squares

References

External links

• Weisstein, Eric W., "Vecten Points", MathWorld.