Type-2 Gumbel distribution
| Parameters |
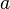 (real) (real) shape (real) shape (real) |
|---|---|
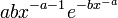 | |
| CDF |
 |
In probability theory, the Type-2 Gumbel probability density function is
for
 .
.
This implies that it is similar to the Weibull distributions, substituting  and
and  . Note however that a positive k (as in the Weibull distribution) would yield a negative a, which is not allowed here as it would yield a negative probability density.
. Note however that a positive k (as in the Weibull distribution) would yield a negative a, which is not allowed here as it would yield a negative probability density.
For  the mean is infinite. For
the mean is infinite. For 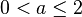 the variance is infinite.
the variance is infinite.
The cumulative distribution function is
The moments ![E[X^k] \,](../I/m/29510905c680e09a5038af0eacb572f1.png) exist for
exist for 
The special case b = 1 yields the Fréchet distribution
Based on The GNU Scientific Library, used under GFDL.