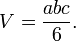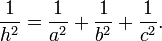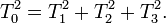Trirectangular tetrahedron
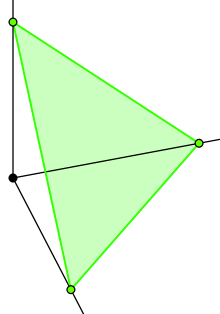
A trirectangular tetrahedron can be constructed by a coordinate octant and a plane crossing all 3 axes away from the origin, like:
x>0
y>0
z>0
and x/a+y/b+z/c<1
x>0
y>0
z>0
and x/a+y/b+z/c<1
In geometry, a trirectangular tetrahedron is a tetrahedron where all three face angles at one vertex are right angles. That vertex is called the right angle of the trirectangular tetrahedron and the face opposite it is called the base. The three edges that meet at the right angle are called the legs and the perpendicular from the right angle to the base is called the altitude of the tetrahedron.
Metric formulas
If the legs have lengths a, b, c, then the trirectangular tetrahedron has the volume
The altitude h satisfies[1]
The area 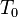 of the base is given by[2]
of the base is given by[2]
De Gua's theorem
Main article: De Gua's theorem
If the area of the base is 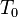 and the areas of the three other (right-angled) faces are
and the areas of the three other (right-angled) faces are 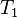 ,
, 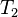 and
and 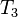 , then
, then
This is a generalization of the Pythagorean theorem to a tetrahedron.
See also
- Disphenoid
- Goursat tetrahedron
- Orthocentric tetrahedron
- Schläfli orthoscheme
- Standard simplex