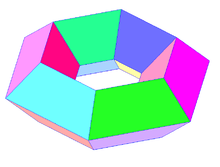
Toroidal polyhedron

The expanded cuboctahedron with rhombic faces excavated has all regular polygon faces as a genus 11 toroidal polyhedron.

A polyhedral torus can be constructed to approximate a torus surface, from a net of quadrilateral faces, like this 6x4 example.

In geometry, a toroidal polyhedron is a polyhedron which is also a toroid (a g-holed torus), having a topological genus of 1 or greater.^[1]

Non-self-intersecting toroidal polyhedra are embedded toroids, while self-intersecting toroidal polyhedra are toroidal as abstract polyhedra, which can be verified by their Euler characteristic (0 or less), and their self-intersecting realization in Euclidean 3-space is a polyhedral immersion.

If a toroidal polyhedron is non-orientable then it cannot be embedded in 3-space, for example the Klein bottle is a non-orientable toroid of Euler characteristic 0. If the toroid has an odd-valued Euler characteristic then it cannot be embedded, however the Klein bottle demonstrates that the converse is not true.

Stewart toroids

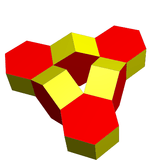
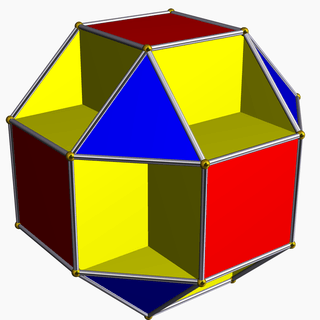
A special category of toroidal polyhedra are constructed exclusively by regular polygon faces, no intersections, and a further restriction that adjacent faces may not exist in the same plane. These are called Stewart toroids, named after Professor Bonnie Stewart who explored their existence.

Stewart also defined them as quasi-convex toroidal polyhedra if the convex hull created no new edges (i.e. the holes can be filled by single planar polygons).

Stewart toroids by augmentation

Six hexagonal prisms	Four square cupolae 8 tetrahedra	Eight octahedra

Császár and Szilassi polyhedra

The Császár polyhedron is a seven-vertex toroidal polyhedron with 21 edges and 14 triangular faces. It and the tetrahedron are the only known polyhedra in which every possible line segment connecting two vertices forms an edge of the polyhedron. Its dual, the Szilassi polyhedron, has seven hexagonal faces that are all adjacent to each other.

The Császár polyhedron has the fewest possible vertices of any toroidal polyhedron, and the Szilassi polyhedron has the fewest possible faces of any toroidal polyhedron.

Self-intersecting tori

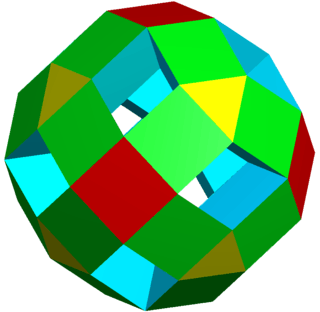
Octahemioctahedron	Cubicuboctahedron	Great dodecahedron

Allowing faces to intersect produces toroidal polyhedra that are hard to recognize except by determining their Euler characteristic: χ = 2(1 − g). Such polyhedra are toroidal as abstract polyhedra, and their self-intersecting realization in Euclidean 3-space is a polyhedral immersion.

For example:

Crown polyhedra

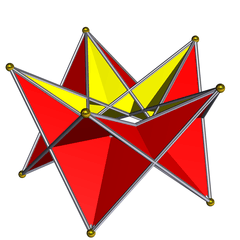
Pentagonal stephanoid. This stephanoid has pentagonal dihedral symmetry and has the same vertices as the uniform pentagonal prism.

A crown polyhedron or stephanoid is a toroidal polyhedron which is also noble, being both isogonal (equal vertices) and isohedral (equal faces). Crown polyhedra are self-intersecting and topologically self-dual.^[2]

References

Notes

↑ Stewart (1964).
↑ Grünbaum, B.; Polyhedra with hollow faces, Proc. NATO-ASI Conf. on polytopes: abstract, convex and computational, Toronto 1983, Ed. Bisztriczky, T. Et Al., Kluwer Academic (1994), pp. 43–70.

Bibliography

B. M. Stewart, Adventures Among the Toroids (1970) ISBN 978-0-686-11936-4