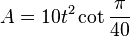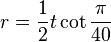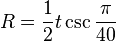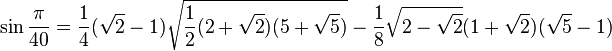Tetracontagon

Regular tetracontagon

A regular tetracontagon
Type Regular polygon
Edges and vertices 40
Schläfli symbol {40}
t{20}
Coxeter diagram
Symmetry group Dihedral (D40), order 2×40
Internal angle (degrees) 171°
Dual polygon self
Properties convex, cyclic, equilateral, isogonal, isotoxal

In geometry, a tetracontagon or tessaracontagon is a forty-sided polygon.[1][2] The sum of any tetracontagon's interior angles is 6840 degrees.

A regular tetracontagon is represented by Schläfli symbol {40} and can also be constructed as a quasiregular truncated icosagon, t{20}, which alternates two types of edges.

Regular tetracontagon properties

One interior angle in a regular tetracontagon is 171°, meaning that one exterior angle would be 9°.

The area of a regular tetracontagon is (with t = edge length)

A = 10t^2 \cot \frac{\pi}{40}

and its inradius is

r = \frac{1}{2}t \cot \frac{\pi}{40}

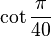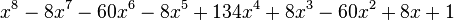The factor \cot \frac{\pi}{40} is a root of the octic equation x^{8} - 8x^{7} - 60x^{6} - 8x^{5} + 134x^{4} + 8x^{3} - 60x^{2} + 8x + 1.

The circumradius of a regular tetracontagon is

R = \frac{1}{2}t \csc \frac{\pi}{40}

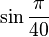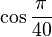A regular tetracontagon is constructible using a compass and straightedge.[3] As a truncated icosagon, it can be constructed by an edge-bisection of a regular icosagon. This means that the values of \sin \frac{\pi}{40} and \cos \frac{\pi}{40} may be expressed in radicals as follows:

\sin \frac{\pi}{40} = \frac{1}{4}(\sqrt{2}-1)\sqrt{\frac{1}{2}(2+\sqrt{2})(5+\sqrt{5})}-\frac{1}{8}\sqrt{2-\sqrt{2}}(1+\sqrt{2})(\sqrt{5}-1)
\cos \frac{\pi}{40} = \frac{1}{8}(\sqrt{2}-1)\sqrt{2+\sqrt{2}}(\sqrt{5}-1)+\frac{1}{4}(1+\sqrt{2})\sqrt{\frac{1}{2}(2-\sqrt{2})(5+\sqrt{5})}

Tetracontagram

A tetracontagram is a 40-sided star polygon. There are 7 regular forms given by Schläfli symbols {40/3}, {40/7}, {40/9}, {40/11}, {40/13}, {40/17}, and {40/19}, and 12 compound star figures with the same vertex configuration.

Regular star polygons {40/k}
Picture
{40/3}
{40/7}
{40/9}
{40/11}
{40/13}
{40/17}
{40/19}
Interior angle 153° 117° 99° 81° 63° 27° 9°
Regular compound polygons
Picture
{40/2}=2{20}
{40/4}=4{10}
{40/5}=5{8}
{40/6}=2{20/3}
{40/8}=8{5}
{40/10}=10{4}
Interior angle 162° 144° 135° 126° 108° 90°
Picture
{40/12}=4{10/3}
{40/14}=2{20/7}
{40/15}=5{8/3}
{40/16}=8{5/2}
{40/18}=2{20/9}
{40/20}=20{2}
Interior angle 72° 54° 45° 36° 18° 0°

Many isogonal tetracontagrams can also be constructed as deeper truncations of the regular icosagon {20} and icosagrams {20/3}, {20/7}, and {20/9}. These also create four quasitruncations: t{20/11}={40/11}, t{20/13}={40/13}, t{20/17}={40/17}, and t{20/19}={40/19}. Some of the isogonal tetracontagrams are depicted below, as a truncation sequence with endpoints t{20}={40} and t{20/19}={40/19}.[4]


t{20}={40}
t{20/19}={40/19}

References

  1. ↑ Gorini, Catherine A. (2009), The Facts on File Geometry Handbook, Infobase Publishing, p. 165, ISBN 9781438109572.
  2. ↑ The New Elements of Mathematics: Algebra and Geometry by Charles Sanders Peirce (1976), p.298
  3. ↑ Constructible Polygon
  4. ↑ The Lighter Side of Mathematics: Proceedings of the Eugène Strens Memorial Conference on Recreational Mathematics and its History, (1994), Metamorphoses of polygons, Branko Grünbaum