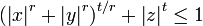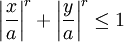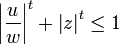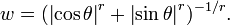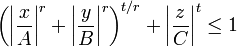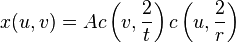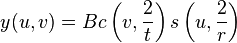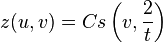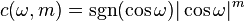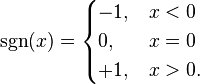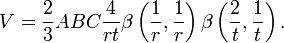Superellipsoid

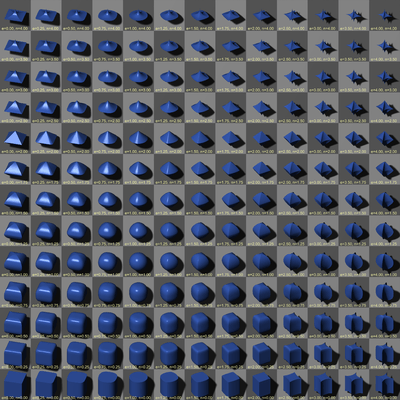
Superellipsoid collection with exponent parameters, created using POV-Ray. Here, e = 2/r, and n = 2/t (equivalently, r = 2/e and t = 2/n).[1] The cube, cylinder, sphere, Steinmetz solid, bicone and regular octahedron can all be seen as special cases.

In mathematics, a super-ellipsoid or superellipsoid is a solid whose horizontal sections are super-ellipses (Lamé curves) with the same exponent r, and whose vertical sections through the center are super-ellipses with the same exponent t.

Super-ellipsoids as computer graphics primitives were popularized by Alan H. Barr (who used the name "superquadrics" to refer to both superellipsoids and supertoroids).[2][3] However, while some super-ellipsoids are superquadrics, neither family is contained in the other.

Piet Hein's supereggs are special cases of super-ellipsoids.

Formulas

Basic shape

The basic super-ellipsoid is defined by the implicit equation

\left( \left|x\right|^{r} + \left|y\right|^{r} \right)^{t/r} + \left|z\right|^{t} \leq 1

The parameters r and t are positive real numbers that control the amount of flattening at the tips and at the equator. Note that the formula becomes a special case of the superquadric's equation if (and only if) t = r.

Any "parallel of latitude" of the superellipsoid (a horizontal section at any constant z between -1 and +1) is a Lamé curve with exponent r, scaled by a = (1 - \left|z\right|^{t})^{1/t}:

\left|\frac{x}{a}\right|^{r} + \left|\frac{y}{a}\right|^{r} \leq 1

Any "meridian of longitude" (a section by any vertical plane through the origin) is a Lamé curve with exponent t, stretched horizontally by a factor w that depends on the sectioning plane. Namely, if x = u cos θ and y = u sin θ, for a fixed θ, then

\left|\frac{u}{w}\right|^t + \left|z\right|^t \leq 1

where

w = (\left|\cos \theta\right|^r + \left|\sin\theta\right|^r)^{-1/r}.

In particular, if r is 2, the horizontal cross-sections are circles, and the horizontal stretching w of the vertical sections is 1 for all planes. In that case, the super-ellipsoid is a solid of revolution, obtained by rotating the Lamé curve with exponent t around the vertical axis.

The basic shape above extends from −1 to +1 along each coordinate axis. The general super-ellipsoid is obtained by scaling the basic shape along each axis by factors A, B, C, the semi-diameters of the resulting solid. The implicit equation is

\left( \left|\frac{x}{A}\right|^r + \left|\frac{y}{B}\right|^r \right)^{t/r} + \left|\frac{z}{C}\right|^{t} \leq 1

Setting r = 2, t = 2.5, A = B = 3, C = 4 one obtains Piet Hein's superegg.

The general superellipsoid has a parametric representation in terms of surface parameters -π/2 < v < π/2, -π < u < π [3]

x(u,v) = A c\left(v,\frac{2}{t}\right) c\left(u,\frac{2}{r}\right)
y(u,v) = B c\left(v,\frac{2}{t}\right) s\left(u,\frac{2}{r}\right)
z(u,v) = C s\left(v,\frac{2}{t}\right)

where the auxiliary functions are

c(\omega,m) = \sgn(\cos \omega) |\cos \omega|^m
s(\omega,m) = \sgn(\sin \omega) |\sin \omega|^m

and the sign function sgn(x) is

\sgn(x) = \begin{cases} -1, & x < 0 \\ 0, & x = 0 \\ +1, & x > 0 . \end{cases}

The volume inside this surface can be expressed in terms of beta functions, β(m,n) = Γ(m)Γ(n)/Γ(m + n), as

V = \frac23 A B C \frac{4}{r t} \beta \left( \frac{1}{r},\frac{1}{r} \right) \beta \left(\frac{2}{t},\frac{1}{t} \right).

See also

References

  1. ↑ http://www.povray.org/documentation/view/3.6.1/285/
  2. ↑ Barr, A.H. (January 1981), Superquadrics and Angle-Preserving Transformations. IEEE_CGA vol. 1 no. 1, pp. 11–23
  3. ↑ 3.0 3.1 Barr, A.H. (1992), Rigid Physically Based Superquadrics. Chapter III.8 of Graphics Gems III, edited by D. Kirk, pp. 137–159

External links