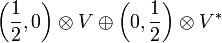Super-Poincaré algebra
In theoretical physics, a super-Poincaré algebra is an extension of the Poincaré algebra to incorporate supersymmetry, a relation between bosons and fermions. They are examples of supersymmetry algebras (without central charges or internal symmetries), and are Lie superalgebras. Thus a super-Poincaré algebra is a Z2 graded vector space with a graded Lie bracket such that the even part is a Lie algebra containing the Poincaré algebra, and the odd part is built from spinors on which there is an anticommutation relation with values in the even part.
The simplest supersymmetric extension of the Poincaré algebra contains two Weyl spinors with the following anti-commutation relation:
and all other anti-commutation relations between the Qs and Ps vanish.[1] In the above expression 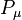 are the generators of translation and
are the generators of translation and 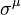 are the Pauli matrices.
are the Pauli matrices.
To do this in full form it is easy to introduce the General Relativity metric. The Pauli and Dirac matrices should then depend on the metric 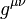 as:
as:
and
This then gives the full algebra[2]
![[ Q_\alpha , P^\mu ] = 0](../I/m/6bea1c3b2c606d90a90e46a5f7edf9f6.png)
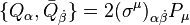
with the addition of the normal Poincaré algebra. It is a closed algebra since all Jacobi identities are satisfied and can have since explicit matrix representations. Following this line of reasoning will lead to Supergravity.
SUSY in 3 + 1 Minkowski spacetime
In 3+1 Minkowski spacetime, the Haag-Lopuszanski-Sohnius theorem states that the SUSY algebra with N spinor generators is as follows.
The even part of the star Lie superalgebra is the direct sum of the Poincaré algebra and a reductive Lie algebra B (such that its self-adjoint part is the tangent space of a real compact Lie group). The odd part of the algebra would be
where  and
and 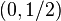 are specific representations of the Poincaré algebra. Both components are conjugate to each other under the * conjugation. V is an N-dimensional complex representation of B and V* is its dual representation. The Lie bracket for the odd part is given by a symmetric equivariant pairing {.,.} on the odd part with values in the even part. In particular, its reduced intertwiner from
are specific representations of the Poincaré algebra. Both components are conjugate to each other under the * conjugation. V is an N-dimensional complex representation of B and V* is its dual representation. The Lie bracket for the odd part is given by a symmetric equivariant pairing {.,.} on the odd part with values in the even part. In particular, its reduced intertwiner from ![[(\frac{1}{2},0)\otimes V]\otimes[(0,\frac{1}{2})\otimes V^*]](../I/m/cefb9f3b9be0c1e9de3db69639e4ef7f.png) to the ideal of the Poincaré algebra generated by translations is given as the product of a nonzero intertwiner from
to the ideal of the Poincaré algebra generated by translations is given as the product of a nonzero intertwiner from 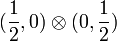 to (1/2,1/2). The "contraction intertwiner" from
to (1/2,1/2). The "contraction intertwiner" from 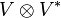 to the trivial representation and the reduced intertwiner from
to the trivial representation and the reduced intertwiner from ![[(\frac{1}{2},0)\otimes V]\otimes [(\frac{1}{2},0)\otimes V]](../I/m/36a06a398d46592a1557256ecb851055.png) is the product of a (antisymmetric) intertwiner from (1/2,0) squared to (0,0) and an antisymmetric intertwiner A from
is the product of a (antisymmetric) intertwiner from (1/2,0) squared to (0,0) and an antisymmetric intertwiner A from  to B. * conjugate it to get the corresponding case for the other half.
to B. * conjugate it to get the corresponding case for the other half.
N = 1
B is now 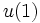 (called R-symmetry) and V is the 1D representation of
(called R-symmetry) and V is the 1D representation of 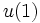 with "charge" 1. A (the intertwiner defined above) would have to be zero since it is antisymmetric.
with "charge" 1. A (the intertwiner defined above) would have to be zero since it is antisymmetric.
Actually, there are two versions of N=1 SUSY, one without the 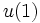 (i.e. B is zero-dimensional) and the other with
(i.e. B is zero-dimensional) and the other with 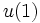 .
.
N = 2
B is now 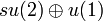 and V is the 2D doublet representation of
and V is the 2D doublet representation of  with a zero
with a zero 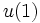 "charge". Now, A is a nonzero intertwiner to the
"charge". Now, A is a nonzero intertwiner to the 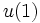 part of B.
part of B.
Alternatively, V could be a 2D doublet with a nonzero 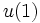 "charge". In this case, A would have to be zero.
"charge". In this case, A would have to be zero.
Yet another possibility would be to let B be 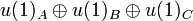 . V is invariant under
. V is invariant under 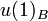 and
and 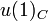 and decomposes into a 1D rep with
and decomposes into a 1D rep with  charge 1 and another 1D rep with charge -1. The intertwiner A would be complex with the real part mapping to
charge 1 and another 1D rep with charge -1. The intertwiner A would be complex with the real part mapping to 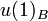 and the imaginary part mapping to
and the imaginary part mapping to 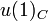 .
.
Or we could have B being 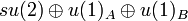 with V being the doublet rep of
with V being the doublet rep of  with zero
with zero 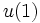 charges and A being a complex intertwiner with the real part mapping to
charges and A being a complex intertwiner with the real part mapping to  and the imaginary part to
and the imaginary part to 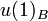 .
.
This doesn't even exhaust all the possibilities. We see that there is more than one N = 2 supersymmetry; likewise, the SUSYs for N > 2 are also not unique (in fact, it only gets worse).
N = 3
It is theoretically allowed, but the multiplet structure becomes automatically the same with that of an N=4 supersymmetric theory. So it is less often discussed compared to N=1,2,4 versions.
N = 4
This is the maximal number of supercharges in a theory without gravity.
SUSY in various dimensions
In 0 + 1, 2 + 1, 3 + 1, 4 + 1, 6 + 1, 7 + 1, 8 + 1, 10 + 1 dimensions, etc., a SUSY algebra is classified by a positive integer N.
In 1 + 1, 5 + 1, 9 + 1 dimensions, etc., a SUSY algebra is classified by two nonnegative integers (M, N), at least one of which is nonzero. M represents the number of left-handed SUSYs and N represents the number of right-handed SUSYs.
The reason of this has to do with the reality conditions of the spinors.
Hereafter d = 9 means d = 8 + 1 in Minkowski signature, etc. The structure of supersymmetry algebra is mainly determined by the number of the fermionic generators, that is the number N times the real dimension of the spinor in d dimensions. It is because one can obtain a supersymmetry algebra of lower dimension easily from that of higher dimensionality by the use of dimensional reduction.
d = 11
The only example is the N = 1 supersymmetry with 32 supercharges.
d = 10
From d = 11, N = 1 susy, one obtains N = (1, 1) nonchiral susy algebra, which is also called the type IIA supersymmetry. There is also N = (2, 0) susy algebra, which is called the type IIB supersymmetry. Both of them have 32 supercharges.
N = (1, 0) susy algebra with 16 supercharges is the minimal susy algebra in 10 dimensions. It is also called the type I supersymmetry. Type IIA / IIB / I Superstring theory has the susy algebra of the corresponding name. The supersymmetry algebra for the heterotic superstrings is that of type I .
References
- ↑ Aitchison, Ian J R. "Supersymmetry and the MSSM: An Elementary Introduction". arXiv:hep-ph/0505105.
- ↑ P. van Nieuwenhuizen, Phys. Rep. 68, 189 (1981)
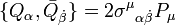

![\sigma^{\mu \nu}=\frac{i}{2}[ \gamma^\mu,\gamma^\nu ]](../I/m/b6e623d6e50c7c346fd8a474f174d4d0.png)
![[ M^{\mu \nu} , Q_\alpha ] = \frac{1}{2} ( \sigma^{\mu \nu})_\alpha^\beta Q_\beta](../I/m/570599eb037eaf8b928577f7b607cf14.png)