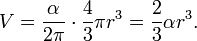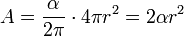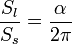Spherical wedge

A spherical wedge with radius r and angle of the wedge α

In geometry, a spherical wedge or ungula is a portion of a ball bounded by two plane semidisks and a spherical lune (termed the wedge's base). The angle between the radii lying within the bounding semidisks is the dihedral angle of the wedge α. If AB is a semidisk that forms a ball when completely revolved about the z-axis, revolving AB only through a given α produces a spherical wedge of the same angle α.[1] Beman (2008)[2] remarks that "a spherical wedge is to the sphere of which it is a part as the angle of the wedge is to a perigon."[A] A spherical wedge of α = π radians (180°) is called a hemisphere, while a spherical wedge of α = 2π radians (360°) constitutes a complete ball.

The volume of a spherical wedge can be intuitively related to the AB definition in that while the volume of a ball of radius r is given by 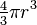\tfrac{4}{3} \pi r^3, the volume a spherical wedge of the same radius r is given by[3]

V = \frac{\alpha}{2\pi} \cdot \frac{4}{3} \pi r^3 = \frac{2}{3} \alpha r^3.

Extrapolating the same principle and considering that the surface area of a sphere is given by 4\pi r^2, it can be seen that the surface area of the lune corresponding to the same wedge is given by[A]

A = \frac{\alpha}{2\pi} \cdot 4 \pi r^2 = 2 \alpha r^2

Hart (2009)[3] states that the "volume of a spherical wedge is to the volume of the sphere as the number of degrees in the [angle of the wedge] is to 360".[A] Hence, and through derivation of the spherical wedge volume formula, it can be concluded that, if 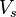V_s is the volume of the sphere and 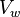V_w is the volume of a given spherical wedge,

\frac{V_w}{V_s} = \frac{\alpha}{2\pi}

Also, if Sl is the area of a given wedge's lune, and Ss is the area of the wedge's sphere,[4][A]

\frac{S_l}{S_s} = \frac{\alpha}{2\pi}

See also

Notes

A. ^ A distinction is sometimes drawn between the terms "sphere" and "ball", where a sphere is regarded as being merely the outer surface of a solid ball. It is common to use the terms interchangeably, as the commentaries of both Beman (2008) and Hart (2008) do.

References

  1. ↑ P. Morton (1830). Geometry, plane, solid, and spherical, in six books. Baldwin and Cradock. p. 180.
  2. ↑ D. W. Beman (2008). New Plane and Solid Geometry. BiblioBazaar, LLC. p. 338. ISBN 0-554-44701-0.
  3. ↑ 3.0 3.1 C. A. Hart (2009). Solid Geometry. BiblioBazaar, LLC. p. 465. ISBN 1-103-11804-8.
  4. ↑ E. A. Avallone, T. Baumeister, A. Sadegh, L. S. Marks (2006). Marks' standard handbook for mechanical engineers. McGraw-Hill Professional. p. 43. ISBN 0-07-142867-4.