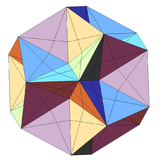
Small triambic icosahedron

Small triambic icosahedron
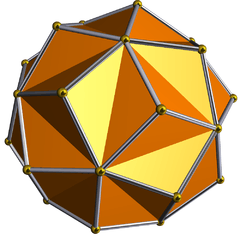Type	Dual uniform polyhedron
Index	DU30, 2/59, W26
Elements (As a star polyhedron)	F = 20, E = 60 V = 32 (χ = −8)
Symmetry group	icosahedral (Ih)
Dual polyhedron	small ditrigonal icosidodecahedron
Stellation diagram Stellation core Convex hull Icosahedron Pentakis dodecahedron

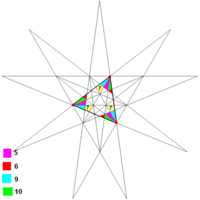
In geometry, the small triambic icosahedron is the dual to the uniform small ditrigonal icosidodecahedron. It is composed of 20 intersecting isogonal hexagon faces. It has 60 edges and 32 vertices, and Euler characteristic of −8. Its external surface also represents the B stellation of the icosahedron.

If the intersected hexagonal faces are divided and new edges created, this figure becomes the triakis icosahedron. The descriptive name triakis icosahedron represents a topological construction starting with an icosahedron and attaching tetrahedra to each face. With the proper pyramid height this figure becomes a Catalan solid by the same name and the dual of the truncated dodecahedron.

The nonconvex uniform polyhedra great stellated dodecahedron and great dodecahedron, as viewed as surface topologies, can also be constructed as icosahedron with pyramids, the first with much taller pyramids, and the second with inverted ones.

It is also a uniform dual, and is the dual of the small ditrigonal icosidodecahedron. Other uniform duals which are also stellations of the icosahedron are the medial triambic icosahedron and the great triambic icosahedron.

As a stellation

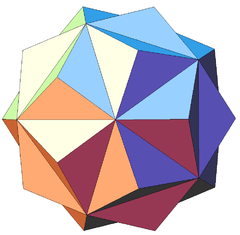
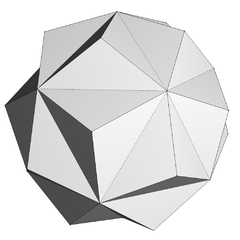
This figure is also the first stellation of the icosahedron, and given as Wenninger model index 26.

This stellation is a popular subject for construction in modular origami, often made of thirty Sonobe units.

References

• Wenninger, Magnus (1974). Polyhedron Models. Cambridge University Press. ISBN 0-521-09859-9.  (p. 46, Model W₂₆, triakis icosahedron)
• Wenninger, Magnus (1983). Dual Models. Cambridge University Press. ISBN 0-521-54325-8.  (pp. 42–46, dual to uniform polyhedron W₇₀)
• Coxeter, Harold Scott MacDonald; Du Val, P.; Flather, H. T.; Petrie, J. F. (1999). The fifty-nine icosahedra (3rd ed.). Tarquin. ISBN 978-1-899618-32-3. MR 676126.  (1st Edn University of Toronto (1938))
• H.S.M. Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8, 3.6 6.2 Stellating the Platonic solids, pp.96-104

External links

• Weisstein, Eric W., "Small triambic icosahedron", MathWorld.

Notable stellations of the icosahedron
Regular	Uniform duals			Regular compounds			Regular star	Others
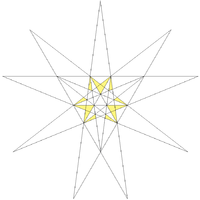
(Convex) icosahedron	Small triambic icosahedron	Medial triambic icosahedron	Great triambic icosahedron	Compound of five octahedra	Compound of five tetrahedra	Compound of ten tetrahedra	Great icosahedron	Excavated dodecahedron	Final stellation
The stellation process on the icosahedron creates a number of related polyhedra and compounds with icosahedral symmetry.