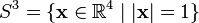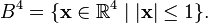Slice knot

A slice knot is a type of mathematical knot. In knot theory, a "knot" means an embedded circle in the 3-sphere

S^3 = \{\mathbf{x}\in \mathbb{R}^4 \mid |\mathbf{x}|=1 \}

and that the 3-sphere can be thought of as the boundary of the four-dimensional ball

B^4 = \{\mathbf{x}\in \mathbb{R}^4 \mid |\mathbf{x}|\leq 1 \}.

A knot K\subset S^3 is slice if it bounds a nicely embedded disk D in the 4-ball.[1]

What is meant by "nicely embedded" depends on the context, and there are different terms for different kinds of slice knots. If D is smoothly embedded in B4, then K is said to be smoothly slice. If K is only locally flat (which is weaker), then K is said to be topologically slice.

Every ribbon knot is smoothly slice. An old question of Fox asks whether every slice knot is actually a ribbon knot.[2]

The signature of a slice knot is zero.[3]

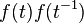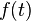The Alexander polynomial of a slice knot factors as a product f(t)f(t^{-1}) where f(t) is some integral Laurent polynomial.[3] This is known as the Fox–Milnor condition.[4]

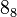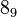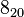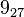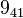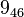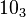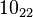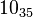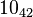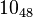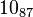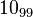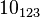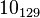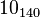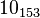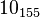The following is a list of all slice knots with 10 or fewer crossings; it was compiled using the Knot Atlas: 61, 8_8, 8_9, 8_{20}, 9_{27}, 9_{41}, 9_{46}, 10_3, 10_{22}, 10_{35}, 10_{42}, 10_{48}, 10_{75}, 10_{87}, 10_{99}, 10_{123}, 10_{129}, 10_{137}, 10_{140}, 10_{153} and 10_{155}.

References

  1. ↑ Lickorish, W. B. Raymond (1997), An Introduction to Knot Theory, Graduate Texts in Mathematics 175, Springer, p. 86, ISBN 9780387982540.
  2. ↑ Gompf, Robert E.; Scharlemann, Martin; Thompson, Abigail (2010), "Fibered knots and potential counterexamples to the property 2R and slice-ribbon conjectures", Geometry & Topology 14 (4): 2305–2347, doi:10.2140/gt.2010.14.2305, MR 2740649.
  3. ↑ 3.0 3.1 Lickorish (1997), p. 90.
  4. ↑ Banagl, Markus; Vogel, Denis (2010), The Mathematics of Knots: Theory and Application, Contributions in Mathematical and Computational Sciences 1, Springer, p. 61, ISBN 9783642156373.

See also