Skorokhod problem
In probability theory, a the Skorokhod problem is the problem of solving a stochastic differential equation with a reflecting boundary condition.[1]
The problem is named after Anatoliy Skorokhod who first published the solution to a stochastic differential equation for a reflecting Brownian motion.[2][3][4]
Problem statement
The classic version of the problem states[5] that given a càdlàg process {X(t), t ≥ 0} and an M-matrix R, then stochastic processes {W(t), t ≥ 0} and {Z(t), t ≥ 0} are said to solve the Skorokhod problem if for all non-negative t values,
- W(t) = X(t) + R Z(t) ≥ 0
- Z(0) = 0 and dZ(t) ≥ 0
-
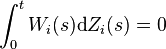 .
.
The matrix R is often known as the reflection matrix, Z(t) as the reflected process and W(t) as the regulator process.
References
- ↑ Lions, P. L.; Sznitman, A. S. (1984). "Stochastic differential equations with reflecting boundary conditions". Communications on Pure and Applied Mathematics 37 (4): 511. doi:10.1002/cpa.3160370408.
- ↑ Skorokhod, A. V. (1961). "Stochastic equations for diffusion processes in a bounded region 1". Theor. Veroyatnost. i Primenen. 6: 264–274.
- ↑ Skorokhod, A. V. (1962). "Stochastic equations for diffusion processes in a bounded region 2". Theor. Veroyatnost. i Primenen. 7: 3–23.
- ↑ Tanaka, Hiroshi (1979). "Stochastic differential equations with reflecting boundary condition in convex regions". Hiroshima Math. J. 9 (1): 163–177.
- ↑ Haddad, J. P.; Mazumdar, R. R.; Piera, F. J. (2010). "Pathwise comparison results for stochastic fluid networks". Queueing Systems 66 (2): 155. doi:10.1007/s11134-010-9187-9.