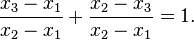Siegel identity

In mathematics, Siegel's identity refers to one of two formulae that are used in the resolution of Diophantine equations.

Statement

The first formula is

\frac{x_3 - x_1}{x_2 - x_1} + \frac{x_2 - x_3}{x_2 - x_1} = 1 .

The second is

\frac{x_3 - x_1}{x_2 - x_1} \cdot\frac{t - x_2}{t - x_3} + \frac{x_2 - x_3}{x_2 - x_1} \cdot \frac{t - x_1}{t - x_3} = 1 .

The identities are used in translating Diophantine problems connected with integral points on hyperelliptic curves into S-unit equations.

Baker, Alan (1975). Transcendental Number Theory. Cambridge University Press. p. 40. ISBN 0-521-20461-5. Zbl 0297.10013.
Baker, Alan; Wüstholz, Gisbert (2007). Logarithmic Forms and Diophantine Geometry. New Mathematical Monographs 9. Cambridge University Press. p. 53. ISBN 978-0-521-88268-2. Zbl 1145.11004.
Kubert, Daniel S.; Lang, Serge (1981). Modular Units. Grundlehren der Mathematischen Wissenschaften 244. ISBN 0-387-90517-0.
Lang, Serge (1978). Elliptic Curves: Diophantine Analysis. Grundlehren der mathematischen Wissenschaften 231. Springer-Verlag. ISBN 0-387-08489-4.
Smart, N. P. (1998). The Algorithmic Resolution of Diophantine Equations. London Mathematical Society Student Texts 41. Cambridge University Press. pp. 36–37. ISBN 0-521-64633-2.