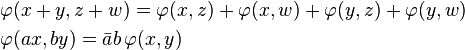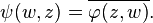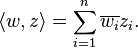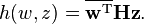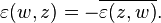Sesquilinear form

In mathematics, a sesquilinear form is a generalization of a bilinear form that, in turn, is a generalization of the concept of the dot product of Euclidean space. A bilinear form is linear in each of its arguments, but a sesquilinear form allows one of the arguments to be "twisted" in a semilinear manner, thus the name; which originates from the Latin numerical prefix sesqui- meaning "one and a half". The basic concept of the dot product — producing a scalar from a pair of vectors — can be generalized by allowing a broader range of scalar values and, perhaps simultaneously, by widening the definition of what a vector is.

A motivating special case is a sesquilinear form on a complex vector space, $V$ . This is a map $V \times V \to C$ that is linear in one argument and "twists" the linearity of other argument by complex conjugation (referred to as being antilinear in the other argument). This case arises naturally in mathematical physics applications. Another important case allows the scalars to come from any field and the twist is provided by a field automorphism. Many authors assume that this automorphism is an involution (has order two) to stay in analogy with the complex case, but others prove this property when introducing Hermitian forms.

An application in projective geometry requires that the scalars come from a division ring (skewfield), $K$ , and this means that the "vectors" should be replaced by elements of a $K$ -module. In a very general setting, sesquilinear forms can be defined over $R$ -modules for arbitrary rings $R$ .

Convention

Conventions differ as to which argument should be linear. We shall take the first to be linear, as is common in the mathematical literature, except in the section devoted to sesquilinear forms on complex vector spaces. There we use the other convention and take the first argument to be conjugate-linear (i.e. antilinear) and the second to be linear. This is the convention used mostly by mathematical physicists^[1] and originates in Dirac's bra–ket notation in quantum mechanics.

Complex vector spaces

Over a complex vector space $V$ a map $φ : V \times V \to C$ is sesquilinear if

\begin{align} &\varphi(x + y, z + w) = \varphi(x, z) + \varphi(x, w) + \varphi(y, z) + \varphi(y, w)\\ &\varphi(a x, b y) = \bar a b\,\varphi(x,y)\end{align}

for all $x, y, z, w \in V$ and all $a, b 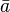\in C$ . $\bar a$ is the complex conjugate of a.

A complex sesquilinear form can also be viewed as a complex bilinear map

\bar V \times V \to \mathbf{C}

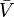where $\bar V$ is the complex conjugate vector space to $V$ . By the universal property of tensor products these are in one-to-one correspondence with complex linear maps

\bar V \otimes V \to \mathbf{C}.

For a fixed $z$ in $V$ the map $w \mapsto φ (z, w)$ is a linear functional on $V$ (i.e. an element of the dual space $V *$ ). Likewise, the map 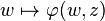$w \mapsto \varphi(w,z)$ is a conjugate-linear functional on $V$ .

Given any complex sesquilinear form $φ$ on $V$ we can define a second complex sesquilinear form $ψ$ via the conjugate transpose:

\psi(w,z) = \overline{\varphi(z,w)}.

In general, $ψ$ and $φ$ will be different. If they are the same then $φ$ is said to be Hermitian. If they are negatives of one another, then $φ$ is said to be skew-Hermitian. Every sesquilinear form can be written as a sum of a Hermitian form and a skew-Hermitian form.

Geometric motivation

Bilinear forms are to squaring ( $z 2$ ), what complex sesquilinear forms are to Euclidean norm (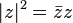 $|z|^2 = \bar z z$ ).

The norm associated to a complex sesquilinear form is invariant under multiplication by complex numbers of unit norm (elements of the complex unit circle), while the norm associated to a bilinear form is equivariant (with respect to squaring). Bilinear forms are algebraically more natural, while sesquilinear forms are geometrically more natural.

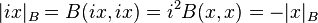If $B$ is a bilinear form on a complex vector space and $|x|_B := B(x,x)$ is the associated norm, then $|ix|_B = B(ix,ix) = i^{2}B(x,x) = -|x|_B$ .

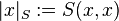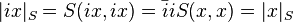By contrast, if $S$ is a sesquilinear form on a complex vector space and $|x|_S := S(x,x)$ is the associated norm, then $|ix|_S = S(ix,ix)=\bar i i S(x,x) = |x|_S$ .

Hermitian form

The term Hermitian form may also refer to a different concept than that explained below: it may refer to a certain differential form on a Hermitian manifold.

A complex Hermitian form (also called a symmetric sesquilinear form), is a sesquilinear form $h : V \times V \to C$ such that

h(w,z) = \overline{h(z, w)}.

The standard Hermitian form on $C n$ is given (again, using the "physics" convention of linearity in the second and conjugate linearity in the first variable) by

\langle w,z \rangle = \sum_{i=1}^n \overline{w_i} z_i.

More generally, the inner product on any complex Hilbert space is a Hermitian form.

A vector space with a Hermitian form $(V, h)$ is called a Hermitian space.

If $V$ is a finite-dimensional complex vector space, then relative to any basis ${e i}$ of $V$ , a complex Hermitian form is represented by a Hermitian matrix $H$ :

h(w,z) = \overline{\mathbf{w}^\mathrm{T}} \mathbf{Hz}.

The components of $H$ are given by $H ij = h (e i, e j)$ .

The quadratic form associated to a complex Hermitian form

Q (z) = h (z, z)

is always real. Actually, one can show that a complex sesquilinear form is Hermitian iff the associated quadratic form is real for all $z \in V$ .

Skew-Hermitian form

A complex skew-Hermitian form (also called an antisymmetric sesquilinear form), is a complex sesquilinear form $ε : V \times V \to C$ such that

\varepsilon(w,z) = -\overline{\varepsilon(z, w)}.

Every complex skew-Hermitian form can be written as $i$ times a Hermitian form.

If $V$ is a finite-dimensional complex vector space, then relative to any basis { $e i$ } of $V$ , a complex skew-Hermitian form is represented by a skew-Hermitian matrix $A$ :

\varepsilon(w,z) = \overline{\mathbf{w}}^\mathrm{T} \mathbf{Az}.

The quadratic form associated to a complex skew-Hermitian form

Q (z) = ε (z, z)

is always pure imaginary.

Over arbitrary fields

On a vector space $V$ defined over an arbitrary field $F$ having a distinguished automorphism $σ$ of order two (an involution), a map $φ : V \times V \to F$ is sesquilinear if

\begin{align} &\varphi(x + y, z + w) = \varphi(x, z) + \varphi(x, w) + \varphi(y, z) + \varphi(y, w)\\ &\varphi(c x, d y) = c d^{\sigma} \varphi(x,y) = c \,\sigma (d) \,\varphi(x,y)\end{align}

for all $x, y, z, w \in V$ and all $c, d \in F$ . Recall the convention of having the first argument linear and notice the use of the "transformation exponential notation" $t \mapsto t σ$ .

If the automorphism $σ = id$ then the sesquilinear form is a bilinear form.

A sesquilinear form $φ$ is said to be $σ$ -Hermitian if

\varphi(x,y) = \varphi(y,x)^{\sigma}

for all $x, y \in V$ . It follows from this definition that $φ (x, x)$ always lies in the fixed field of $σ$ . In the bilinear case ( $σ = id$ ) these forms are called symmetric.

A sesquilinear form can also be viewed as an $F$ -bilinear map

V \times V^* \to F

where $V *$ is the dual space of $V$ .

In projective geometry

A generalization called a semi-bilinear form was used by Reinhold Baer to characterize linear manifolds (projective spaces) that are dual to each other in chapter 4 of his book Linear Algebra and Projective Geometry (1952).^[2] For a skewfield F and A a vector space over F he requires:^[3]

A pair consisting of an anti-automorphism α of the skewfield

F

and a function

f : A \times A \to F

satisfying

for all

a, b, c \in A

f (a + b, c) = f (a, c) + f (b, c)

f (a, b + c) = f (a, b) + f (a, c)

, and

for all

t \in F

and

x, y \in A

f (tx, y) = tf (x, y)

f (x, ty) = f (x, y) t α

(page 101)

(The "transformation exponential notation"

t \mapsto t α

is adopted in group theory literature.)

Baer calls such a form an α-form over A. The complex sesquilinear form described above has complex conjugation for α. When α is the identity, then f is a bilinear form.

In the algebraic structure called a *-ring the anti-automorphism is denoted by * and forms are constructed as indicated for α. Special constructions such as skew-symmetric bilinear forms, Hermitian forms, and skew-Hermitian forms are all considered in the broader context.

Particularly in L-theory, one also sees the term $ε$ -symmetric form, where $ε = \pm1$ , to refer to both symmetric and skew-symmetric forms.

Notes

↑ footnote 1 in Anthony Knapp Basic Algebra (2007) pg. 255
↑ Baer, Reinhold (2005) [1952], Linear Algebra and Projective Geometry, Dover, ISBN 978-0-486-44565-6
↑ Baer uses the term (not necessarily commutative) field for division ring (= skewfield) and refers to A as an abelian group linear over F meaning an F-module (more colloquially known as a vector space over a skewfield)

References

Gruenberg, K.W.; Weir, A.J. (1977), Linear Geometry (2nd ed.), Springer, ISBN 0-387-90227-9
Hazewinkel, Michiel, ed. (2001), "Sesquilinear form", Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4