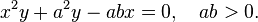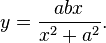Serpentine curve

For serpentine shapes in architecture and design, see Serpentine shape.

A serpentine curve is a curve whose equation is of the form

x^2y+a^2y-abx=0, \quad ab > 0.

Equivalently, it has a parametric representation

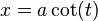x=a\cot(t)

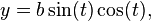
y=b\sin (t)\cos(t),

or functional representation

y=\frac{abx}{x^2+a^2}.

History

Serpentine curves were studied by L'Hôpital and Huygens, and named and classified by Newton.

The serpentine curve for a = b = 1.