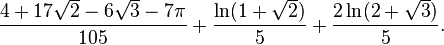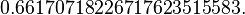Robbins constant

In geometry, Robbins' constant, named after David P. Robbins, is the average distance between two points selected at random within a unit cube. Robbins' constant can be expressed as [1]

\frac{4+17\sqrt2-6\sqrt3-7\pi}{105} + \frac{\ln(1+\sqrt2)}{5} + \frac{2\ln(2+\sqrt3)}{5}.

Its numerical value is approximately [2]

0.66170718226717623515583.

References

  1. ↑ Robbins, David P.; Bolis, Theodore S. (1978), "Average distance between two points in a box (solution to elementary problem E2629)", American Mathematical Monthly 85 (4): 277–278, doi:10.2307/2321177.
  2. ↑ Plouffe, Simon, "The Robbins constant", Miscellaneous Mathematical Constants