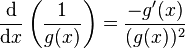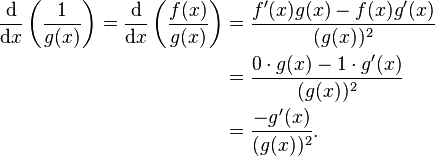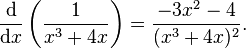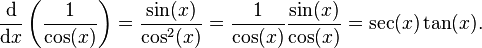Reciprocal rule

In calculus, the reciprocal rule is a shorthand method of finding the derivative of a function that is the reciprocal of a differentiable function, without using the quotient rule or chain rule.

The reciprocal rule states that the derivative of 1/g(x) is given by

\frac{\mathrm d}{\mathrm dx}\left(\frac{1}{g(x)}\right) = \frac{- g'(x)}{(g(x))^2}

where g(x) ≠ 0.

Proof

From the quotient rule

The reciprocal rule is derived from the quotient rule, with the numerator f(x) = 1. Then:

\begin{align} \frac{\mathrm d}{\mathrm dx}\left(\frac{1}{g(x)}\right) = \frac{\mathrm d}{\mathrm dx}\left(\frac{f(x)}{g(x)}\right) & = \frac{f'(x)g(x) - f(x)g'(x)}{(g(x))^2}\\ {} & = \frac{0\cdot g(x) - 1\cdot g'(x)}{(g(x))^2}\\ {} & = \frac{- g'(x)}{(g(x))^2}.\end{align}

From the chain rule

It is also possible to derive the reciprocal rule from the chain rule, by a process very much like that of the derivation of the quotient rule. One thinks of 1/g(x) as being the function 1/x composed with the function g(x). The result then follows by application of the chain rule.

Examples

The derivative of 1/(x3+4x) is:

\frac{\mathrm d}{\mathrm dx}\left(\frac{1}{x^3 + 4x}\right) = \frac{-3x^2 - 4}{(x^3 + 4x)^2}.

The derivative of 1/cos(x) (when cos(x) ≠ 0) is:

\frac{\mathrm d}{\mathrm dx} \left(\frac{1}{\cos(x) }\right) = \frac{\sin(x)}{\cos^2(x)} = \frac{1}{\cos(x)} \frac{\sin(x)}{\cos(x)} = \sec(x)\tan(x).

For more general examples, see the derivative article.

See also