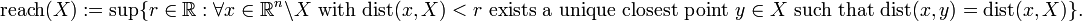Reach (mathematics)

In mathematics, the reach of a subset of Euclidean space Rⁿ is a real number that roughly describes how curved the boundary of the set is.

Definition

Let X be a subset of Rⁿ. Then reach of X is defined as

\text{reach}(X) := \sup \{r \in \mathbb{R}: \forall x \in \mathbb{R}^n\setminus X\text{ with }{\rm dist}(x,X) < r \text{ exists a unique closest point }y \in X\text{ such that }{\rm dist}(x,y)= {\rm dist}(x,X)\}.

Shapes that have reach infinity:

The graph of ƒ(x) = |x| has reach zero.

A circle of radius r has reach r.

Federer, Herbert (1969), Geometric measure theory, Die Grundlehren der mathematischen Wissenschaften 153, New York: Springer-Verlag New York Inc., pp. xiv+676, ISBN 978-3-540-60656-7, MR 0257325, Zbl 0176.00801